Online courses directory (418)

The course is an introduction to linear and discrete optimization - an important part of computational mathematics with a wide range of applications in many areas of everyday life.

This course covers mathematical topics in algebra and trigonometry and is designed to prepare students to enroll for a first semester course in single variable calculus.

Calculating derivatives. Power rule. Product and quotient rules. Chain Rule. Implicit differentiation. Derivatives of common functions. Newton Leibniz and Usain Bolt. Slope of a line secant to a curve. Slope of a secant line example 1. Slope of a secant line example 2. Slope of a secant line example 3. Approximating instantaneous rate of change word problem. Approximating equation of tangent line word problem. Slope of secant lines. Derivative as slope of a tangent line. Tangent slope as limiting value of secant slope example 1. Tangent slope as limiting value of secant slope example 2. Tangent slope as limiting value of secant slope example 3. Tangent slope is limiting value of secant slope. Calculating slope of tangent line using derivative definition. Derivatives 1. The derivative of f(x)=x^2 for any x. Formal and alternate form of the derivative. Formal and alternate form of the derivative for ln x. Formal and alternate form of the derivative example 1. The formal and alternate form of the derivative. Interpreting slope of a curve exercise. Recognizing slope of curves. Calculus: Derivatives 1. Calculus: Derivatives 2. Derivative Intuition Module. Derivative intuition. Graphs of functions and their derivatives example 1. Where a function is not differentiable. Identifying a function's derivative example. Figuring out which function is the the derivative. Graphs of functions and their derivatives. Intuitively drawing the derivative of a function. Intuitively drawing the antiderivative of a function. Visualizing derivatives exercise. Visualizing derivatives. Power Rule. Is the power rule reasonable. Derivative properties and polynomial derivatives. Power rule. Proof: d/dx(x^n). Proof: d/dx(sqrt(x)). Power rule introduction. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Chain rule introduction. Chain rule definition and example. Chain rule with triple composition. Chain rule for derivative of 2^x. Derivative of log with arbitrary base. Chain rule 1. Extreme Derivative Word Problem (advanced). The Chain Rule. Chain Rule Examples. Even More Chain Rule. More examples using multiple rules. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Applying the product rule for derivatives. Product rule for more than two functions. Product rule. Quotient rule from product rule. Quotient rule for derivative of tan x. Quotient rule. Using the product rule and the chain rule. Product Rule. Quotient rule and common derivatives. Equation of a tangent line. Implicit differentiation. Showing explicit and implicit differentiation give same result. Implicit derivative of (x-y)^2 = x + y + 1. Implicit derivative of y = cos(5x - 3y). Implicit derivative of (x^2+y^2)^3 = 5x^2y^2. Finding slope of tangent line with implicit differentiation. Implicit derivative of e^(xy^2) = x - y. Derivative of x^(x^x). Implicit differentiation. Proof: d/dx(ln x) = 1/x. Proof: d/dx(e^x) = e^x. Proofs of derivatives of ln(x) and e^x. Newton Leibniz and Usain Bolt. Slope of a line secant to a curve. Slope of a secant line example 1. Slope of a secant line example 2. Slope of a secant line example 3. Approximating instantaneous rate of change word problem. Approximating equation of tangent line word problem. Slope of secant lines. Derivative as slope of a tangent line. Tangent slope as limiting value of secant slope example 1. Tangent slope as limiting value of secant slope example 2. Tangent slope as limiting value of secant slope example 3. Tangent slope is limiting value of secant slope. Calculating slope of tangent line using derivative definition. Derivatives 1. The derivative of f(x)=x^2 for any x. Formal and alternate form of the derivative. Formal and alternate form of the derivative for ln x. Formal and alternate form of the derivative example 1. The formal and alternate form of the derivative. Interpreting slope of a curve exercise. Recognizing slope of curves. Calculus: Derivatives 1. Calculus: Derivatives 2. Derivative Intuition Module. Derivative intuition. Graphs of functions and their derivatives example 1. Where a function is not differentiable. Identifying a function's derivative example. Figuring out which function is the the derivative. Graphs of functions and their derivatives. Intuitively drawing the derivative of a function. Intuitively drawing the antiderivative of a function. Visualizing derivatives exercise. Visualizing derivatives. Power Rule. Is the power rule reasonable. Derivative properties and polynomial derivatives. Power rule. Proof: d/dx(x^n). Proof: d/dx(sqrt(x)). Power rule introduction. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Chain rule introduction. Chain rule definition and example. Chain rule with triple composition. Chain rule for derivative of 2^x. Derivative of log with arbitrary base. Chain rule 1. Extreme Derivative Word Problem (advanced). The Chain Rule. Chain Rule Examples. Even More Chain Rule. More examples using multiple rules. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Applying the product rule for derivatives. Product rule for more than two functions. Product rule. Quotient rule from product rule. Quotient rule for derivative of tan x. Quotient rule. Using the product rule and the chain rule. Product Rule. Quotient rule and common derivatives. Equation of a tangent line. Implicit differentiation. Showing explicit and implicit differentiation give same result. Implicit derivative of (x-y)^2 = x + y + 1. Implicit derivative of y = cos(5x - 3y). Implicit derivative of (x^2+y^2)^3 = 5x^2y^2. Finding slope of tangent line with implicit differentiation. Implicit derivative of e^(xy^2) = x - y. Derivative of x^(x^x). Implicit differentiation. Proof: d/dx(ln x) = 1/x. Proof: d/dx(e^x) = e^x. Proofs of derivatives of ln(x) and e^x.

This free online training explores complex numbers and equations, polynomial equations, conics, advanced trigonometry, differentiation, antiderivatives, and vectors in 2- and 3-space. This course is both an ideal study-aid for students to improve their skills in their spare time or for anyone interested in exploring the world of mathematics.

The Advanced Mathematics Upper-Secondary 2 course completes our suit of upper-secondary maths. This free online course covers differential equations, kinematics, vector calculus and dynamics. This course is suitable for maths students, and for anyone interested in exploring the world of mathematics. <br /><br />

Indefinite integral as anti-derivative. Definite integral as area under a curve. Integration by parts. U-substitution. Trig substitution. Antiderivatives and indefinite integrals. Indefinite integrals of x raised to a power. Antiderivative of hairier expression. Basic trig and exponential antiderivatives. Antiderivative of x^-1. Simple Riemann approximation using rectangles. Generalizing a left Riemann sum with equally spaced rectangles. Rectangular and trapezoidal Riemann approximations. Trapezoidal approximation of area under curve. Riemann sums and integrals. Deriving integration by parts formula. Antiderivative of xcosx using integration by parts. Integral of ln x. Integration by parts twice for antiderivative of (x^2)(e^x). Integration by parts of (e^x)(cos x). U-substitution. U-substitution example 2. U-substitution Example 3. U-substitution with ln(x). Doing u-substitution twice (second time with w). U-substitution and back substitution. U-substitution with definite integral. (2^ln x)/x Antiderivative Example. Another u-substitution example. Riemann sums and integrals. Intuition for Second Fundamental Theorem of Calculus. Evaluating simple definite integral. Definite integrals and negative area. Area between curves. Area between curves with multiple boundaries. Challenging definite integration. Introduction to definite integrals. Definite integrals (part II). Definite Integrals (area under a curve) (part III). Definite Integrals (part 4). Definite Integrals (part 5). Definite integral with substitution. Introduction to trig substitution. Another substitution with x=sin (theta). Integrals: Trig Substitution 1. Trig and U substitution together (part 1). Trig and U substitution together (part 2). Trig substitution with tangent. Integrals: Trig Substitution 2. Integrals: Trig Substitution 3 (long problem). Fundamental theorem of calculus. Applying the fundamental theorem of calculus. Swapping the bounds for definite integral. Both bounds being a function of x. Proof of Fundamental Theorem of Calculus. Connecting the first and second fundamental theorems of calculus. Introduction to improper integrals. Improper integral with two infinite bounds. Divergent improper integral. Antiderivatives and indefinite integrals. Indefinite integrals of x raised to a power. Antiderivative of hairier expression. Basic trig and exponential antiderivatives. Antiderivative of x^-1. Simple Riemann approximation using rectangles. Generalizing a left Riemann sum with equally spaced rectangles. Rectangular and trapezoidal Riemann approximations. Trapezoidal approximation of area under curve. Riemann sums and integrals. Deriving integration by parts formula. Antiderivative of xcosx using integration by parts. Integral of ln x. Integration by parts twice for antiderivative of (x^2)(e^x). Integration by parts of (e^x)(cos x). U-substitution. U-substitution example 2. U-substitution Example 3. U-substitution with ln(x). Doing u-substitution twice (second time with w). U-substitution and back substitution. U-substitution with definite integral. (2^ln x)/x Antiderivative Example. Another u-substitution example. Riemann sums and integrals. Intuition for Second Fundamental Theorem of Calculus. Evaluating simple definite integral. Definite integrals and negative area. Area between curves. Area between curves with multiple boundaries. Challenging definite integration. Introduction to definite integrals. Definite integrals (part II). Definite Integrals (area under a curve) (part III). Definite Integrals (part 4). Definite Integrals (part 5). Definite integral with substitution. Introduction to trig substitution. Another substitution with x=sin (theta). Integrals: Trig Substitution 1. Trig and U substitution together (part 1). Trig and U substitution together (part 2). Trig substitution with tangent. Integrals: Trig Substitution 2. Integrals: Trig Substitution 3 (long problem). Fundamental theorem of calculus. Applying the fundamental theorem of calculus. Swapping the bounds for definite integral. Both bounds being a function of x. Proof of Fundamental Theorem of Calculus. Connecting the first and second fundamental theorems of calculus. Introduction to improper integrals. Improper integral with two infinite bounds. Divergent improper integral.

Topics covered in a traditional college level introductory microeconomics course. Production Possibilities Frontier. Opportunity Cost. Increasing Opportunity Cost. Allocative Efficiency and Marginal Benefit. Economic Growth through Investment. Comparative Advantage Specialization and Gains from Trade. Comparative Advantage and Absolute Advantage. Law of Demand. Price of Related Products and Demand. Changes in Income, Population, or Preferences. Normal and Inferior Goods. Inferior Goods Clarification. Law of Supply. Factors Affecting Supply. Market Equilibrium. Changes in Market Equilibrium. Price Elasticity of Demand. More on Elasticity of Demand. Perfect Inelasticity and Perfect Elasticity of Demand. Constant Unit Elasticity. Total Revenue and Elasticity. More on Total Revenue and Elasticity. Cross Elasticity of Demand. Elasticity of Supply. Elasticity and Strange Percent Changes. Demand Curve as Marginal Benefit Curve. Consumer Surplus Introduction. Total Consumer Surplus as Area. Producer Surplus. Rent Control and Deadweight Loss. Minimum Wage and Price Floors. Taxation and Dead Weight Loss. Percentage Tax on Hamburgers. Taxes and Perfectly Inelastic Demand. Taxes and Perfectly Elastic Demand. Marginal Utility. Equalizing Marginal Utility per Dollar Spent. Deriving Demand Curve from Tweaking Marginal Utility per Dollar. Budget Line. Optimal Point on Budget Line. Types of Indifference Curves. Economic Profit vs Accounting Profit. Depreciation and Opportunity Cost of Capital. Fixed, Variable, and Marginal Cost.. Visualizing Average Costs and Marginal Costs as Slope. Marginal Cost and Average Total Cost. Marginal Revenue and Marginal Cost. Marginal Revenue Below Average Total Cost. Long Term Supply Curve and Economic Profit. Perfect Competition. Monopoly Basics. Review of Revenue and Cost Graphs for a Monopoly. Monopolist Optimizing Price (part 1)- Total Revenue.. Monopolist Optimizing Price (part 2)- Marginal Revenue. Monopolist Optimizing Price (part 3)- Dead Weight Loss.avi. Optional Calculus Proof to Show that MR has Twice Slope of Demand. Oligopolies and Monopolistic Competition. Monopolistic Competition and Economic Profit. Oligopolies, Duopolies, Collusion, and Cartels. Prisoners' Dilemma and Nash Equilibrium. More on Nash Equilibrium. Why Parties to Cartels Cheat. Game Theory of Cheating Firms. Negative Externalities. Taxes for Factoring in Negative Externalities. Positive Externalities. Tragedy of the Commons. First Degree Price Discrimination. A Firm's Marginal Product Revenue Curve. How Many People to Hire Given the MPR curve. Adding Demand Curves.

Parameterizing a surface. Surface integrals. Stokes' theorem. Introduction to Parametrizing a Surface with Two Parameters. Determining a Position Vector-Valued Function for a Parametrization of Two Parameters. Partial Derivatives of Vector-Valued Functions. Introduction to the Surface Integral. Example of calculating a surface integral part 1. Example of calculating a surface integral part 2. Example of calculating a surface integral part 3. Surface Integral Example Part 1 - Parameterizing the Unit Sphere. Surface Integral Example Part 2 - Calculating the Surface Differential. Surface Integral Example Part 3 - The Home Stretch. Surface Integral Ex2 part 1 - Parameterizing the Surface. Surface Integral Ex2 part 2 - Evaluating Integral. Surface Integral Ex3 part 1 - Parameterizing the Outside Surface. Surface Integral Ex3 part 2 - Evaluating the Outside Surface. Surface Integral Ex3 part 3 - Top surface. Surface Integral Ex3 part 4 - Home Stretch. Conceptual Understanding of Flux in Three Dimensions. Constructing a unit normal vector to a surface. Vector representation of a Surface Integral. Stokes' Theorem Intuition. Green's and Stokes' Theorem Relationship. Orienting Boundary with Surface. Orientation and Stokes. Conditions for Stokes Theorem. Stokes Example Part 1. Part 2 Parameterizing the Surface. Stokes Example Part 3 - Surface to Double Integral. Stokes Example Part 4 - Curl and Final Answer. Evaluating Line Integral Directly - Part 1. Evaluating Line Integral Directly - Part 2. Stokes' Theorem Proof Part 1. Stokes' Theorem Proof Part 2. Stokes' Theorem Proof Part 3. Stokes' Theorem Proof Part 4. Stokes' Theorem Proof Part 5. Stokes' Theorem Proof Part 6. Stokes' Theorem Proof Part 7. Introduction to Parametrizing a Surface with Two Parameters. Determining a Position Vector-Valued Function for a Parametrization of Two Parameters. Partial Derivatives of Vector-Valued Functions. Introduction to the Surface Integral. Example of calculating a surface integral part 1. Example of calculating a surface integral part 2. Example of calculating a surface integral part 3. Surface Integral Example Part 1 - Parameterizing the Unit Sphere. Surface Integral Example Part 2 - Calculating the Surface Differential. Surface Integral Example Part 3 - The Home Stretch. Surface Integral Ex2 part 1 - Parameterizing the Surface. Surface Integral Ex2 part 2 - Evaluating Integral. Surface Integral Ex3 part 1 - Parameterizing the Outside Surface. Surface Integral Ex3 part 2 - Evaluating the Outside Surface. Surface Integral Ex3 part 3 - Top surface. Surface Integral Ex3 part 4 - Home Stretch. Conceptual Understanding of Flux in Three Dimensions. Constructing a unit normal vector to a surface. Vector representation of a Surface Integral. Stokes' Theorem Intuition. Green's and Stokes' Theorem Relationship. Orienting Boundary with Surface. Orientation and Stokes. Conditions for Stokes Theorem. Stokes Example Part 1. Part 2 Parameterizing the Surface. Stokes Example Part 3 - Surface to Double Integral. Stokes Example Part 4 - Curl and Final Answer. Evaluating Line Integral Directly - Part 1. Evaluating Line Integral Directly - Part 2. Stokes' Theorem Proof Part 1. Stokes' Theorem Proof Part 2. Stokes' Theorem Proof Part 3. Stokes' Theorem Proof Part 4. Stokes' Theorem Proof Part 5. Stokes' Theorem Proof Part 6. Stokes' Theorem Proof Part 7.

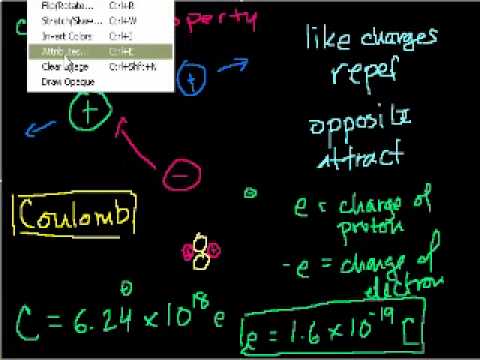
Electrostatics (part 1): Introduction to Charge and Coulomb's Law. Electrostatics (part 2). Proof (Advanced): Field from infinite plate (part 1). Proof (Advanced): Field from infinite plate (part 2). Electric Potential Energy. Electric Potential Energy (part 2-- involves calculus). Voltage. Capacitance. Circuits (part 1). Circuits (part 2). Circuits (part 3). Circuits (part 4). Cross product 1. Cross Product 2. Cross Product and Torque. Introduction to Magnetism. Magnetism 2. Magnetism 3. Magnetism 4. Magnetism 5. Magnetism 6: Magnetic field due to current. Magnetism 7. Magnetism 8. Magnetism 9: Electric Motors. Magnetism 10: Electric Motors. Magnetism 11: Electric Motors. Magnetism 12: Induced Current in a Wire. The dot product. Dot vs. Cross Product. Calculating dot and cross products with unit vector notation. Electrostatics (part 1): Introduction to Charge and Coulomb's Law. Electrostatics (part 2). Proof (Advanced): Field from infinite plate (part 1). Proof (Advanced): Field from infinite plate (part 2). Electric Potential Energy. Electric Potential Energy (part 2-- involves calculus). Voltage. Capacitance. Circuits (part 1). Circuits (part 2). Circuits (part 3). Circuits (part 4). Cross product 1. Cross Product 2. Cross Product and Torque. Introduction to Magnetism. Magnetism 2. Magnetism 3. Magnetism 4. Magnetism 5. Magnetism 6: Magnetic field due to current. Magnetism 7. Magnetism 8. Magnetism 9: Electric Motors. Magnetism 10: Electric Motors. Magnetism 11: Electric Motors. Magnetism 12: Induced Current in a Wire. The dot product. Dot vs. Cross Product. Calculating dot and cross products with unit vector notation.

The second part of our intermediate math course continues our free online maths suite of courses. It covers binomial, normal and hypergeometric distribution, discrete random variables, and integration. This course is ideal for students preparing for an exam, or for those wanting to refresh their knowledge of mathematics.

This free online course introduces you to the mathematics of probability, chance and the analysis of data. The course begins by introducing data collection and analysis, graphs and frequency distribution. The course examines chance in the context of gambling, odds and probability. The course is of interest to anybody who needs to analyse mathematical data and is particularly valuable to students studying for exams.

This free online course is the first of our Upper-Secondary Mathematics suite of courses. It covers mathematical analysis, including univariate statistics and data, bivariate statistics, correlation, regression, residual analysis, non-linear data, and seasonal movements. This course is suitable for students of maths, especially those preparing for examinations. It is also suitable for those looking to refresh their knowledge of Mathematics. <br />

Relationship between angular velocity and speed. Why Distance is Area under Velocity-Time Line. Introduction to Vectors and Scalars. Calculating Average Velocity or Speed. Solving for Time. Displacement from Time and Velocity Example. Acceleration. Balanced and Unbalanced Forces. Unbalanced Forces and Motion. Newton's First Law of Motion. Newton's First Law of Motion Concepts. Newton's First Law of Motion. Newton's Second Law of Motion. Newton's Third Law of Motion. Airbus A380 Take-off Time. Airbus A380 Take-off Distance. Average Velocity for Constant Acceleration. Acceleration of Aircraft Carrier Takeoff. Race Cars with Constant Speed Around Curve. Introduction to Gravity. Mass and Weight Clarification. Gravity for Astronauts in Orbit. Would a Brick or Feather Fall Faster. Deriving Displacement as a Function of Time, Acceleration and Initial Velocity. Plotting Projectile Displacement, Acceleration, and Velocity. Projectile Height Given Time. Deriving Max Projectile Displacement Given Time. Impact Velocity From Given Height. Visualizing Vectors in 2 Dimensions. Projectile at an Angle. Different Way to Determine Time in Air. Launching and Landing on Different Elevations. Total Displacement for Projectile. Total Final Velocity for Projectile. Correction to Total Final Velocity for Projectile. Projectile on an Incline. Unit Vectors and Engineering Notation. Clearing the Green Monster at Fenway. Green Monster at Fenway Part 2. Optimal angle for a projectile part 1. Optimal angle for a projectile part 2 - Hangtime. Optimal angle for a projectile part 3 - Horizontal distance as a function of angle (and speed). Optimal angle for a projectile part 4 Finding the optimal angle and distance with a bit of calculus. Slow Sock on Lubricon VI. Normal Forces on Lubricon VI. Normal Force and Contact Force. Normal Force in an Elevator. Inclined Plane Force Components. Ice Accelerating Down an Incline. Force of Friction Keeping the Block Stationary. Correction to Force of Friction Keeping the Block Stationary. Force of Friction Keeping Velocity Constant. Intuition on Static and Kinetic Friction Comparisons. Static and Kinetic Friction Example. Introduction to Tension. Introduction to Tension (Part 2). Tension in an accelerating system and pie in the face. Introduction to Momentum. Momentum: Ice skater throws a ball. 2-dimensional momentum problem. 2-dimensional momentum problem (part 2). Introduction to work and energy. Work and Energy (part 2). Conservation of Energy. Work/Energy problem with Friction. Introduction to mechanical advantage. Mechanical Advantage (part 2). Mechanical Advantage (part 3). Center of Mass. Introduction to Torque. Moments. Moments (part 2). Unit Vector Notation. Unit Vector Notation (part 2). Projectile Motion with Ordered Set Notation. Projectile motion (part 1). Projectile motion (part 2). Projectile motion (part 3). Projectile motion (part 4). Projectile motion (part 5). Centripetal Force and Acceleration Intuition. Visual Understanding of Centripetal Acceleration Formula. Calculus proof of centripetal acceleration formula. Loop De Loop Question. Loop De Loop Answer part 1. Loop De Loop Answer part 2. Acceleration Due to Gravity at the Space Station. Space Station Speed in Orbit. Conservation of angular momentum. Introduction to Newton's Law of Gravitation. Gravitation (part 2). Viewing g as the value of Earth's Gravitational Field Near the Surface. Intro to springs and Hooke's Law. Potential energy stored in a spring. Spring potential energy example (mistake in math). Introduction to Harmonic Motion. Harmonic Motion Part 2 (calculus). Harmonic Motion Part 3 (no calculus).

Exploring a world where both sides aren't equal anymore!. Inequalities Using Multiplication and Division. One-step inequality with multiplication and division example. Constructing and solving one-step inequality. One step inequalities. One-Step inequality involving addition. Inequalities Using Addition and Subtraction. Two-step inequality example. Multi-Step Inequalities. Multi-Step Inequalities 2. Multi-Step Inequalities 3. Multi-step linear inequalities. Interpreting Inequalities. Writing and using inequalities 2. Writing and using inequalities 3. Interpreting and solving linear inequalities. Inequality examples. Compound Inequalities. Compound Inequalities. Compound Inequalities 2. Compound Inequalities 3. Compound Inequalities 4. Compound inequalities. Absolute Value Inequalities. Absolute value inequalities Example 1. Absolute Value Inequalities Example 2. Absolute value inequalities example 3. Writing and using inequalities. Dogs cats and bears in a pet store visual argument. Dogs cats and bears in a pet store analytic argument. Reasoning through inequality expressions. Using expressions to understand relationships. Structure in expressions 1. Inequalities Using Multiplication and Division. One-step inequality with multiplication and division example. Constructing and solving one-step inequality. One step inequalities. One-Step inequality involving addition. Inequalities Using Addition and Subtraction. Two-step inequality example. Multi-Step Inequalities. Multi-Step Inequalities 2. Multi-Step Inequalities 3. Multi-step linear inequalities. Interpreting Inequalities. Writing and using inequalities 2. Writing and using inequalities 3. Interpreting and solving linear inequalities. Inequality examples. Compound Inequalities. Compound Inequalities. Compound Inequalities 2. Compound Inequalities 3. Compound Inequalities 4. Compound inequalities. Absolute Value Inequalities. Absolute value inequalities Example 1. Absolute Value Inequalities Example 2. Absolute value inequalities example 3. Writing and using inequalities. Dogs cats and bears in a pet store visual argument. Dogs cats and bears in a pet store analytic argument. Reasoning through inequality expressions. Using expressions to understand relationships. Structure in expressions 1.

This course analyzes the functions of a complex variable and the calculus of residues. It also covers subjects such as ordinary differential equations, partial differential equations, Bessel and Legendre functions, and the Sturm-Liouville theory.

Solving exponential and radical expressions and equations. Using scientific notation and significant figures. Negative and Positive Exponents. Evaluating exponential expressions. Evaluating exponential expressions 3. Exponent Rules Part 1. Exponent Rules Part 2. Exponent Properties 1. Exponent Properties 2. Exponent Properties 3. Exponent Properties 4. Simplifying rational expression with exponent properties. Exponent Properties 5. Exponent Properties 6. Exponent Properties 7. Simplifying Square Roots. Simplifying Square Roots Comment Response. Radical Equivalent to Rational Exponents. Radical Equivalent to Rational Exponents 2. Radical Expressions with Higher Roots. Adding and Simplifying Radicals. Subtracting and Simplifying Radicals. Multiply and Simplify a Radical Expression 1. Multiply and Simplify a Radical Expression 2. Exponent Properties Involving Products. Rational Exponents and Exponent Laws. Simplifying Cube Roots. More Rational Exponents and Exponent Laws. How to Rationalize a Denominator. Simplifying Expressions with Exponents. Simplifying Expressions with Exponents 2. Simplifying Expressions with Exponents 3. Fractional Exponent Expressions 1. Fractional Exponent Expressions 2. Fractional Exponent Expressions 3. Simplifying expressions with exponents. Solving Radical Equations. Extraneous Solutions to Radical Equations. Solving Radical Equations 1. Solving Radical Equations 2. Solving Radical Equations 3. Applying Radical Equations 1. Applying Radical Equations 2. Applying Radical Equations 3. Radical equations. Negative and Positive Exponents. Evaluating exponential expressions. Evaluating exponential expressions 3. Exponent Rules Part 1. Exponent Rules Part 2. Exponent Properties 1. Exponent Properties 2. Exponent Properties 3. Exponent Properties 4. Simplifying rational expression with exponent properties. Exponent Properties 5. Exponent Properties 6. Exponent Properties 7. Simplifying Square Roots. Simplifying Square Roots Comment Response. Radical Equivalent to Rational Exponents. Radical Equivalent to Rational Exponents 2. Radical Expressions with Higher Roots. Adding and Simplifying Radicals. Subtracting and Simplifying Radicals. Multiply and Simplify a Radical Expression 1. Multiply and Simplify a Radical Expression 2. Exponent Properties Involving Products. Rational Exponents and Exponent Laws. Simplifying Cube Roots. More Rational Exponents and Exponent Laws. How to Rationalize a Denominator. Simplifying Expressions with Exponents. Simplifying Expressions with Exponents 2. Simplifying Expressions with Exponents 3. Fractional Exponent Expressions 1. Fractional Exponent Expressions 2. Fractional Exponent Expressions 3. Simplifying expressions with exponents. Solving Radical Equations. Extraneous Solutions to Radical Equations. Solving Radical Equations 1. Solving Radical Equations 2. Solving Radical Equations 3. Applying Radical Equations 1. Applying Radical Equations 2. Applying Radical Equations 3. Radical equations.

Logarithms. Ex: Converting an exponential to logarithmic statement. Evaluating logarithms. Fancier logarithm expressions. Evaluating logarithms 2. Graphing Logarithmic Functions. Introduction to Logarithms. Introduction to logarithm properties. Introduction to logarithm properties (part 2). Logarithm of a Power. Sum of Logarithms with Same Base. Using Multiple Logarithm Properties to Simplify. Operations with logarithms. Change of Base Formula. Proof: log a + log b = log ab. Proof: A(log B) = log (B^A), log A - log B = log (A/B). Change of base formula proof. Logarithmic Equations. Solving Logarithmic Equations. Solving Logarithmic Equations. Logarithmic Scale. Richter Scale. Natural Logarithm with a Calculator. Calculator for Natural Logarithms. Graphing Natural Logarithm Function. Logarithms. Ex: Converting an exponential to logarithmic statement. Evaluating logarithms. Fancier logarithm expressions. Evaluating logarithms 2. Graphing Logarithmic Functions. Introduction to Logarithms. Introduction to logarithm properties. Introduction to logarithm properties (part 2). Logarithm of a Power. Sum of Logarithms with Same Base. Using Multiple Logarithm Properties to Simplify. Operations with logarithms. Change of Base Formula. Proof: log a + log b = log ab. Proof: A(log B) = log (B^A), log A - log B = log (A/B). Change of base formula proof. Logarithmic Equations. Solving Logarithmic Equations. Solving Logarithmic Equations. Logarithmic Scale. Richter Scale. Natural Logarithm with a Calculator. Calculator for Natural Logarithms. Graphing Natural Logarithm Function.

Advanced Analytic Methods in Science and Engineering is a comprehensive treatment of the advanced methods of applied mathematics. It was designed to strengthen the mathematical abilities of graduate students and train them to think on their own.

Analysis I covers fundamentals of mathematical analysis: metric spaces, convergence of sequences and series, continuity, differentiability, Riemann integral, sequences and series of functions, uniformity, interchange of limit operations.

This course continues from Analysis I (18.100B), in the direction of manifolds and global analysis. The first half of the course covers multivariable calculus. The rest of the course covers the theory of differential forms in n-dimensional vector spaces and manifolds.