Online courses directory (381)

The art of this period is familiar, since the world of the Realists, Impressionists and Post-Impressionists is much like our own. More and more people lived in cities and worked in factories or shops for wages. Scientific and technological advances increased dramatically during this period and although there was dislocation and privation, standards of living increased sharply. In essence, modern mass culture was born. Artists responded sometimes by embracing these radical changes, and at other times by resisting them. Key here is understanding the authority of the various art academies in Europe, which controlled matters related to taste and art, and which were, to some extent, always connected to the government. A small number of artists rebelled against the strictures of the academy, and against the demand for art to tell clear stories for a middle class audience, and formed what we know as the

An Introduction to Contemporary Art. Why is this Art? Andy Warhol, Campbell's Soup Cans. Art as Concept: In Advance of the Broken Arm. Why Is That Important? Looking at Jackson Pollock. Art & Context: Monet's Cliff Walk at Pourville and Malevich's White on White. Representation & Abstraction: Millais's Ophelia and Newman's Vir Heroicus Sublimis. Interpreting Contemporary Art. Big Questions in Modern & Contemporary Art. Matisse, Luxe, calme et volupt

Most people have had the flu virus at least once in their lives, and it

The Art of Our Time. Bacon, Triptych - August 1972. Freud, Standing by the Rags. Diane Arbus, Boy with a Toy Hand Grenade. William Eggleston, Red Ceiling, or Greenwood, Mississippi, 1973. Ed Kienholz and Nancy Reddin Kienholz Useful Art #5: The Western Hotel, 1992. Warhol, Gold Marilyn Monroe. Warhol, Campbell's Soup Cans. Oldenburg, Floor Cake. Lichtenstein, Rouen Cathedral Set V. Gerhard Richter, Betty. Gerhard Richter, The Cage Paintings (1-6). Gerhard Richter, September. Donald Judd, Untitled. Dan Flavin, Untitled (To Donna) II. Smithson, Spiral Jetty. Hesse, Untitled. Hesse, Untitled (Rope Piece), 1970. Chicago, Pasadena Lifesaver, Blue Series, No. 4 & Benglis, Omega. Winsor, #1 Rope. Joseph Beuys, Table with Accumulator. John Baldessari, I Will Not Make Any More Boring Art. Hans Haacke's Seurat's 'Les Poseuses' (small version). Interpreting Contemporary Art. Colescott, Beauty is in the Eye of the Beholder. Sherman, Untitled Film Still #21. Sherrie Levine, Untitled (After Edward Weston, ca. 1925). The Art of Our Time. Bacon, Triptych - August 1972. Freud, Standing by the Rags. Diane Arbus, Boy with a Toy Hand Grenade. William Eggleston, Red Ceiling, or Greenwood, Mississippi, 1973. Ed Kienholz and Nancy Reddin Kienholz Useful Art #5: The Western Hotel, 1992. Warhol, Gold Marilyn Monroe. Warhol, Campbell's Soup Cans. Oldenburg, Floor Cake. Lichtenstein, Rouen Cathedral Set V. Gerhard Richter, Betty. Gerhard Richter, The Cage Paintings (1-6). Gerhard Richter, September. Donald Judd, Untitled. Dan Flavin, Untitled (To Donna) II. Smithson, Spiral Jetty. Hesse, Untitled. Hesse, Untitled (Rope Piece), 1970. Chicago, Pasadena Lifesaver, Blue Series, No. 4 & Benglis, Omega. Winsor, #1 Rope. Joseph Beuys, Table with Accumulator. John Baldessari, I Will Not Make Any More Boring Art. Hans Haacke's Seurat's 'Les Poseuses' (small version). Interpreting Contemporary Art. Colescott, Beauty is in the Eye of the Beholder. Sherman, Untitled Film Still #21. Sherrie Levine, Untitled (After Edward Weston, ca. 1925).

Introduction to Evolution and Natural Selection. Ape Clarification. Intelligent Design and Evolution. Evolution Clarification. Natural Selection and the Owl Butterfly. DNA. Variation in a Species. Introduction to Evolution and Natural Selection. Ape Clarification. Intelligent Design and Evolution. Evolution Clarification. Natural Selection and the Owl Butterfly. DNA. Variation in a Species.

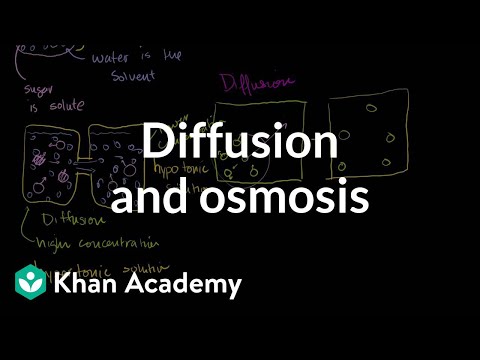
Diffusion and Osmosis. Parts of a cell. Chromosomes, Chromatids, Chromatin, etc.. Mitosis, Meiosis and Sexual Reproduction. Phases of Mitosis. Phases of Meiosis. Embryonic Stem Cells. Cancer. Diffusion and Osmosis. Parts of a cell. Chromosomes, Chromatids, Chromatin, etc.. Mitosis, Meiosis and Sexual Reproduction. Phases of Mitosis. Phases of Meiosis. Embryonic Stem Cells. Cancer.

Introduction to Heredity. Punnett Square Fun. Hardy-Weinberg Principle. Sex-Linked Traits. Genetics 101 Part 1: What are genes?. Genetics 101 Part 2: What are SNPs?. Genetics 101 Part 3: Where do your genes come from?. Genetics 101 Part 4: What are Phenotypes?. Introduction to Heredity. Punnett Square Fun. Hardy-Weinberg Principle. Sex-Linked Traits. Genetics 101 Part 1: What are genes?. Genetics 101 Part 2: What are SNPs?. Genetics 101 Part 3: Where do your genes come from?. Genetics 101 Part 4: What are Phenotypes?.

Taxonomy and the Tree of Life. Species. Bacteria. Viruses. Human Prehistory 101: Prologue. Human Prehistory 101 Part 1: Out of (Eastern) Africa. Human Prehistory 101 Part 2: Weathering The Storm. Human Prehistory 101 Part 3: Agriculture Rocks Our World. Human Prehistory 101: Epilogue. Taxonomy and the Tree of Life. Species. Bacteria. Viruses. Human Prehistory 101: Prologue. Human Prehistory 101 Part 1: Out of (Eastern) Africa. Human Prehistory 101 Part 2: Weathering The Storm. Human Prehistory 101 Part 3: Agriculture Rocks Our World. Human Prehistory 101: Epilogue.

ATP: Adenosine Triphosphate. Introduction to Cellular Respiration. Oxidation and Reduction Review From Biological Point-of-View. Oxidation and Reduction in Cellular Respiration. Krebs / Citric Acid Cycle. Glycolysis. Electron Transport Chain. Oxidative Phosphorylation and Chemiosmosis. ATP: Adenosine Triphosphate. Introduction to Cellular Respiration. Oxidation and Reduction Review From Biological Point-of-View. Oxidation and Reduction in Cellular Respiration. Krebs / Citric Acid Cycle. Glycolysis. Electron Transport Chain. Oxidative Phosphorylation and Chemiosmosis.

ATP: Adenosine Triphosphate. Photosynthesis. Photosynthesis: Light Reactions 1. Photosynthesis: Light Reactions and Photophosphorylation. Photosynthesis: Calvin Cycle. Photorespiration. C-4 Photosynthesis. CAM Plants. ATP: Adenosine Triphosphate. Photosynthesis. Photosynthesis: Light Reactions 1. Photosynthesis: Light Reactions and Photophosphorylation. Photosynthesis: Calvin Cycle. Photorespiration. C-4 Photosynthesis. CAM Plants.

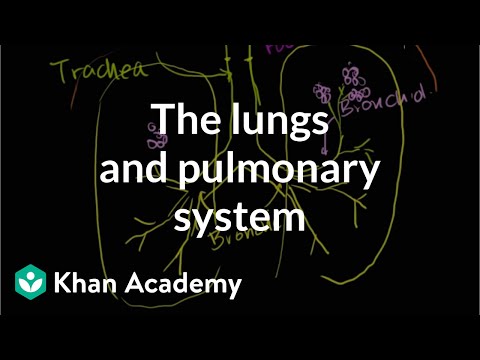
The Lungs and Pulmonary System. Red blood cells. Circulatory System and the Heart. Hemoglobin. Anatomy of a Neuron. Sodium Potassium Pump. Correction to Sodium and Potassium Pump Video. Electrotonic and Action Potentials. Saltatory Conduction in Neurons. Neuronal Synapses (Chemical). Myosin and Actin. Tropomyosin and troponin and their role in regulating muscle contraction. Role of the Sarcoplasmic Reticulum in Muscle Cells. Anatomy of a muscle cell. The Kidney and Nephron. Secondary Active Transport in the Nephron. The Lungs and Pulmonary System. Red blood cells. Circulatory System and the Heart. Hemoglobin. Anatomy of a Neuron. Sodium Potassium Pump. Correction to Sodium and Potassium Pump Video. Electrotonic and Action Potentials. Saltatory Conduction in Neurons. Neuronal Synapses (Chemical). Myosin and Actin. Tropomyosin and troponin and their role in regulating muscle contraction. Role of the Sarcoplasmic Reticulum in Muscle Cells. Anatomy of a muscle cell. The Kidney and Nephron. Secondary Active Transport in the Nephron.

Role of Phagocytes in Innate or Nonspecific Immunity. Types of immune responses: Innate and Adaptive. Humoral vs. Cell-Mediated. B Lymphocytes (B cells). Professional Antigen Presenting Cells (APC) and MHC II complexes. Helper T Cells. Cytotoxic T Cells. Review of B cells, CD4+ T cells and CD8+ T cells. Inflammatory Response. Role of Phagocytes in Innate or Nonspecific Immunity. Types of immune responses: Innate and Adaptive. Humoral vs. Cell-Mediated. B Lymphocytes (B cells). Professional Antigen Presenting Cells (APC) and MHC II complexes. Helper T Cells. Cytotoxic T Cells. Review of B cells, CD4+ T cells and CD8+ T cells. Inflammatory Response.

Calculating derivatives. Power rule. Product and quotient rules. Chain Rule. Implicit differentiation. Derivatives of common functions. Newton Leibniz and Usain Bolt. Slope of a line secant to a curve. Slope of a secant line example 1. Slope of a secant line example 2. Slope of a secant line example 3. Approximating instantaneous rate of change word problem. Approximating equation of tangent line word problem. Slope of secant lines. Derivative as slope of a tangent line. Tangent slope as limiting value of secant slope example 1. Tangent slope as limiting value of secant slope example 2. Tangent slope as limiting value of secant slope example 3. Tangent slope is limiting value of secant slope. Calculating slope of tangent line using derivative definition. Derivatives 1. The derivative of f(x)=x^2 for any x. Formal and alternate form of the derivative. Formal and alternate form of the derivative for ln x. Formal and alternate form of the derivative example 1. The formal and alternate form of the derivative. Interpreting slope of a curve exercise. Recognizing slope of curves. Calculus: Derivatives 1. Calculus: Derivatives 2. Derivative Intuition Module. Derivative intuition. Graphs of functions and their derivatives example 1. Where a function is not differentiable. Identifying a function's derivative example. Figuring out which function is the the derivative. Graphs of functions and their derivatives. Intuitively drawing the derivative of a function. Intuitively drawing the antiderivative of a function. Visualizing derivatives exercise. Visualizing derivatives. Power Rule. Is the power rule reasonable. Derivative properties and polynomial derivatives. Power rule. Proof: d/dx(x^n). Proof: d/dx(sqrt(x)). Power rule introduction. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Chain rule introduction. Chain rule definition and example. Chain rule with triple composition. Chain rule for derivative of 2^x. Derivative of log with arbitrary base. Chain rule 1. Extreme Derivative Word Problem (advanced). The Chain Rule. Chain Rule Examples. Even More Chain Rule. More examples using multiple rules. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Applying the product rule for derivatives. Product rule for more than two functions. Product rule. Quotient rule from product rule. Quotient rule for derivative of tan x. Quotient rule. Using the product rule and the chain rule. Product Rule. Quotient rule and common derivatives. Equation of a tangent line. Implicit differentiation. Showing explicit and implicit differentiation give same result. Implicit derivative of (x-y)^2 = x + y + 1. Implicit derivative of y = cos(5x - 3y). Implicit derivative of (x^2+y^2)^3 = 5x^2y^2. Finding slope of tangent line with implicit differentiation. Implicit derivative of e^(xy^2) = x - y. Derivative of x^(x^x). Implicit differentiation. Proof: d/dx(ln x) = 1/x. Proof: d/dx(e^x) = e^x. Proofs of derivatives of ln(x) and e^x. Newton Leibniz and Usain Bolt. Slope of a line secant to a curve. Slope of a secant line example 1. Slope of a secant line example 2. Slope of a secant line example 3. Approximating instantaneous rate of change word problem. Approximating equation of tangent line word problem. Slope of secant lines. Derivative as slope of a tangent line. Tangent slope as limiting value of secant slope example 1. Tangent slope as limiting value of secant slope example 2. Tangent slope as limiting value of secant slope example 3. Tangent slope is limiting value of secant slope. Calculating slope of tangent line using derivative definition. Derivatives 1. The derivative of f(x)=x^2 for any x. Formal and alternate form of the derivative. Formal and alternate form of the derivative for ln x. Formal and alternate form of the derivative example 1. The formal and alternate form of the derivative. Interpreting slope of a curve exercise. Recognizing slope of curves. Calculus: Derivatives 1. Calculus: Derivatives 2. Derivative Intuition Module. Derivative intuition. Graphs of functions and their derivatives example 1. Where a function is not differentiable. Identifying a function's derivative example. Figuring out which function is the the derivative. Graphs of functions and their derivatives. Intuitively drawing the derivative of a function. Intuitively drawing the antiderivative of a function. Visualizing derivatives exercise. Visualizing derivatives. Power Rule. Is the power rule reasonable. Derivative properties and polynomial derivatives. Power rule. Proof: d/dx(x^n). Proof: d/dx(sqrt(x)). Power rule introduction. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Chain rule introduction. Chain rule definition and example. Chain rule with triple composition. Chain rule for derivative of 2^x. Derivative of log with arbitrary base. Chain rule 1. Extreme Derivative Word Problem (advanced). The Chain Rule. Chain Rule Examples. Even More Chain Rule. More examples using multiple rules. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Applying the product rule for derivatives. Product rule for more than two functions. Product rule. Quotient rule from product rule. Quotient rule for derivative of tan x. Quotient rule. Using the product rule and the chain rule. Product Rule. Quotient rule and common derivatives. Equation of a tangent line. Implicit differentiation. Showing explicit and implicit differentiation give same result. Implicit derivative of (x-y)^2 = x + y + 1. Implicit derivative of y = cos(5x - 3y). Implicit derivative of (x^2+y^2)^3 = 5x^2y^2. Finding slope of tangent line with implicit differentiation. Implicit derivative of e^(xy^2) = x - y. Derivative of x^(x^x). Implicit differentiation. Proof: d/dx(ln x) = 1/x. Proof: d/dx(e^x) = e^x. Proofs of derivatives of ln(x) and e^x.

Indefinite integral as anti-derivative. Definite integral as area under a curve. Integration by parts. U-substitution. Trig substitution. Antiderivatives and indefinite integrals. Indefinite integrals of x raised to a power. Antiderivative of hairier expression. Basic trig and exponential antiderivatives. Antiderivative of x^-1. Simple Riemann approximation using rectangles. Generalizing a left Riemann sum with equally spaced rectangles. Rectangular and trapezoidal Riemann approximations. Trapezoidal approximation of area under curve. Riemann sums and integrals. Deriving integration by parts formula. Antiderivative of xcosx using integration by parts. Integral of ln x. Integration by parts twice for antiderivative of (x^2)(e^x). Integration by parts of (e^x)(cos x). U-substitution. U-substitution example 2. U-substitution Example 3. U-substitution with ln(x). Doing u-substitution twice (second time with w). U-substitution and back substitution. U-substitution with definite integral. (2^ln x)/x Antiderivative Example. Another u-substitution example. Riemann sums and integrals. Intuition for Second Fundamental Theorem of Calculus. Evaluating simple definite integral. Definite integrals and negative area. Area between curves. Area between curves with multiple boundaries. Challenging definite integration. Introduction to definite integrals. Definite integrals (part II). Definite Integrals (area under a curve) (part III). Definite Integrals (part 4). Definite Integrals (part 5). Definite integral with substitution. Introduction to trig substitution. Another substitution with x=sin (theta). Integrals: Trig Substitution 1. Trig and U substitution together (part 1). Trig and U substitution together (part 2). Trig substitution with tangent. Integrals: Trig Substitution 2. Integrals: Trig Substitution 3 (long problem). Fundamental theorem of calculus. Applying the fundamental theorem of calculus. Swapping the bounds for definite integral. Both bounds being a function of x. Proof of Fundamental Theorem of Calculus. Connecting the first and second fundamental theorems of calculus. Introduction to improper integrals. Improper integral with two infinite bounds. Divergent improper integral. Antiderivatives and indefinite integrals. Indefinite integrals of x raised to a power. Antiderivative of hairier expression. Basic trig and exponential antiderivatives. Antiderivative of x^-1. Simple Riemann approximation using rectangles. Generalizing a left Riemann sum with equally spaced rectangles. Rectangular and trapezoidal Riemann approximations. Trapezoidal approximation of area under curve. Riemann sums and integrals. Deriving integration by parts formula. Antiderivative of xcosx using integration by parts. Integral of ln x. Integration by parts twice for antiderivative of (x^2)(e^x). Integration by parts of (e^x)(cos x). U-substitution. U-substitution example 2. U-substitution Example 3. U-substitution with ln(x). Doing u-substitution twice (second time with w). U-substitution and back substitution. U-substitution with definite integral. (2^ln x)/x Antiderivative Example. Another u-substitution example. Riemann sums and integrals. Intuition for Second Fundamental Theorem of Calculus. Evaluating simple definite integral. Definite integrals and negative area. Area between curves. Area between curves with multiple boundaries. Challenging definite integration. Introduction to definite integrals. Definite integrals (part II). Definite Integrals (area under a curve) (part III). Definite Integrals (part 4). Definite Integrals (part 5). Definite integral with substitution. Introduction to trig substitution. Another substitution with x=sin (theta). Integrals: Trig Substitution 1. Trig and U substitution together (part 1). Trig and U substitution together (part 2). Trig substitution with tangent. Integrals: Trig Substitution 2. Integrals: Trig Substitution 3 (long problem). Fundamental theorem of calculus. Applying the fundamental theorem of calculus. Swapping the bounds for definite integral. Both bounds being a function of x. Proof of Fundamental Theorem of Calculus. Connecting the first and second fundamental theorems of calculus. Introduction to improper integrals. Improper integral with two infinite bounds. Divergent improper integral.

Sequences, series and approximating functions. Maclaurin and Taylor series. Sequences and Series (part 1). Sequences and series (part 2). Maclaurin and Taylor Series Intuition. Cosine Taylor Series at 0 (Maclaurin). Sine Taylor Series at 0 (Maclaurin). Taylor Series at 0 (Maclaurin) for e to the x. Euler's Formula and Euler's Identity. Visualizing Taylor Series Approximations. Generalized Taylor Series Approximation. Visualizing Taylor Series for e^x. Error or Remainder of a Taylor Polynomial Approximation. Proof: Bounding the Error or Remainder of a Taylor Polynomial Approximation. Polynomial approximation of functions (part 1). Polynomial approximation of functions (part 2). Approximating functions with polynomials (part 3). Polynomial approximation of functions (part 4). Polynomial approximations of functions (part 5). Polynomial approximation of functions (part 6). Polynomial approximation of functions (part 7). Taylor Polynomials. Sequences and Series (part 1). Sequences and series (part 2). Maclaurin and Taylor Series Intuition. Cosine Taylor Series at 0 (Maclaurin). Sine Taylor Series at 0 (Maclaurin). Taylor Series at 0 (Maclaurin) for e to the x. Euler's Formula and Euler's Identity. Visualizing Taylor Series Approximations. Generalized Taylor Series Approximation. Visualizing Taylor Series for e^x. Error or Remainder of a Taylor Polynomial Approximation. Proof: Bounding the Error or Remainder of a Taylor Polynomial Approximation. Polynomial approximation of functions (part 1). Polynomial approximation of functions (part 2). Approximating functions with polynomials (part 3). Polynomial approximation of functions (part 4). Polynomial approximations of functions (part 5). Polynomial approximation of functions (part 6). Polynomial approximation of functions (part 7). Taylor Polynomials.

Parameterizing a surface. Surface integrals. Stokes' theorem. Introduction to Parametrizing a Surface with Two Parameters. Determining a Position Vector-Valued Function for a Parametrization of Two Parameters. Partial Derivatives of Vector-Valued Functions. Introduction to the Surface Integral. Example of calculating a surface integral part 1. Example of calculating a surface integral part 2. Example of calculating a surface integral part 3. Surface Integral Example Part 1 - Parameterizing the Unit Sphere. Surface Integral Example Part 2 - Calculating the Surface Differential. Surface Integral Example Part 3 - The Home Stretch. Surface Integral Ex2 part 1 - Parameterizing the Surface. Surface Integral Ex2 part 2 - Evaluating Integral. Surface Integral Ex3 part 1 - Parameterizing the Outside Surface. Surface Integral Ex3 part 2 - Evaluating the Outside Surface. Surface Integral Ex3 part 3 - Top surface. Surface Integral Ex3 part 4 - Home Stretch. Conceptual Understanding of Flux in Three Dimensions. Constructing a unit normal vector to a surface. Vector representation of a Surface Integral. Stokes' Theorem Intuition. Green's and Stokes' Theorem Relationship. Orienting Boundary with Surface. Orientation and Stokes. Conditions for Stokes Theorem. Stokes Example Part 1. Part 2 Parameterizing the Surface. Stokes Example Part 3 - Surface to Double Integral. Stokes Example Part 4 - Curl and Final Answer. Evaluating Line Integral Directly - Part 1. Evaluating Line Integral Directly - Part 2. Stokes' Theorem Proof Part 1. Stokes' Theorem Proof Part 2. Stokes' Theorem Proof Part 3. Stokes' Theorem Proof Part 4. Stokes' Theorem Proof Part 5. Stokes' Theorem Proof Part 6. Stokes' Theorem Proof Part 7. Introduction to Parametrizing a Surface with Two Parameters. Determining a Position Vector-Valued Function for a Parametrization of Two Parameters. Partial Derivatives of Vector-Valued Functions. Introduction to the Surface Integral. Example of calculating a surface integral part 1. Example of calculating a surface integral part 2. Example of calculating a surface integral part 3. Surface Integral Example Part 1 - Parameterizing the Unit Sphere. Surface Integral Example Part 2 - Calculating the Surface Differential. Surface Integral Example Part 3 - The Home Stretch. Surface Integral Ex2 part 1 - Parameterizing the Surface. Surface Integral Ex2 part 2 - Evaluating Integral. Surface Integral Ex3 part 1 - Parameterizing the Outside Surface. Surface Integral Ex3 part 2 - Evaluating the Outside Surface. Surface Integral Ex3 part 3 - Top surface. Surface Integral Ex3 part 4 - Home Stretch. Conceptual Understanding of Flux in Three Dimensions. Constructing a unit normal vector to a surface. Vector representation of a Surface Integral. Stokes' Theorem Intuition. Green's and Stokes' Theorem Relationship. Orienting Boundary with Surface. Orientation and Stokes. Conditions for Stokes Theorem. Stokes Example Part 1. Part 2 Parameterizing the Surface. Stokes Example Part 3 - Surface to Double Integral. Stokes Example Part 4 - Curl and Final Answer. Evaluating Line Integral Directly - Part 1. Evaluating Line Integral Directly - Part 2. Stokes' Theorem Proof Part 1. Stokes' Theorem Proof Part 2. Stokes' Theorem Proof Part 3. Stokes' Theorem Proof Part 4. Stokes' Theorem Proof Part 5. Stokes' Theorem Proof Part 6. Stokes' Theorem Proof Part 7.

Overview of the videos, exercises, reports, and Computer Science resources available on Khan Academy, with emphasis on how resources can be used in classrooms. For more information, check out http://www.khanacademy.org/toolkit/ka-resources. For a mapping of Khan Academy content to Common Core standards, check out khanacademy.org/commoncore. Khan Academy overview. Khan Academy reports overview. Khan Academy Exercise Overview. Khan Academy Computer Science in the classroom. Khan Academy overview. Khan Academy reports overview. Khan Academy Exercise Overview. Khan Academy Computer Science in the classroom.

CA Algebra I: Number Properties and Absolute Value. CA Algebra I: Simplifying Expressions. CA Algebra I: Simple Logical Arguments. CA Algebra I: Graphing Inequalities. CA Algebra I: Slope and Y-intercept. CA Algebra I: Systems of Inequalities. CA Algebra I: Simplifying Expressions. CA Algebra I: Factoring Quadratics. CA Algebra I: Completing the Square. CA Algebra I: Quadratic Equation. CA Algebra I: Quadratic Roots. CA Algebra I: Rational Expressions 1. CA Algebra I: Rational Expressions 2. CA Algebra I: Word Problems. CA Algebra I: More Word Problems. CA Algebra I: Functions.

California Standards Test: Algebra II. California Standards Test: Algebra II (Graphing Inequalities). CA Standards: Algebra II (Algebraic Division/Multiplication). CA Standards: Algebra II. Algebra II: Simplifying Polynomials. Algebra II: Imaginary and Complex Numbers. Algebra II: Complex numbers and conjugates. Algebra II: Quadratics and Shifts. Examples: Graphing and interpreting quadratics. Hyperbola and parabola examples. Algebra II: Circles and Logarithms. Algebra II: Logarithms Exponential Growth. Algebra II: Logarithms and more. Algebra II: Functions, Combinatorics. Algebra II: binomial Expansion and Combinatorics. Algebra II: Binomial Expansions, Geometric Series Sum. Algebra II: Functions and Probability. Algebra II: Probability and Statistics. Algebra II: Mean and Standard Deviation.

CA Geometry: deductive reasoning. CA Geometry: Proof by Contradiction. CA Geometry: More Proofs. CA Geometry: Similar Triangles 1. CA Geometry: Similar Triangles 2. CA Geometry: More on congruent and similar triangles. CA Geometry: Triangles and Parallelograms. CA Geometry: Area, Pythagorean Theorem. CA Geometry: Area, Circumference, Volume. CA Geometry: Pythagorean Theorem, Area. CA Geometry: Exterior Angles. CA Geometry: Deducing Angle Measures. CA Geometry: Pythagorean Theorem, Compass Constructions. CA Geometry: Compass Construction. CA Geometry: Basic Trigonometry. CA Geometry: More Trig. CA Geometry: Circle Area Chords Tangent. CA Geometry: Secants and Translations.