Courses tagged with "Diels-Alder" (18)

CA Geometry: deductive reasoning. CA Geometry: Proof by Contradiction. CA Geometry: More Proofs. CA Geometry: Similar Triangles 1. CA Geometry: Similar Triangles 2. CA Geometry: More on congruent and similar triangles. CA Geometry: Triangles and Parallelograms. CA Geometry: Area, Pythagorean Theorem. CA Geometry: Area, Circumference, Volume. CA Geometry: Pythagorean Theorem, Area. CA Geometry: Exterior Angles. CA Geometry: Deducing Angle Measures. CA Geometry: Pythagorean Theorem, Compass Constructions. CA Geometry: Compass Construction. CA Geometry: Basic Trigonometry. CA Geometry: More Trig. CA Geometry: Circle Area Chords Tangent. CA Geometry: Secants and Translations.

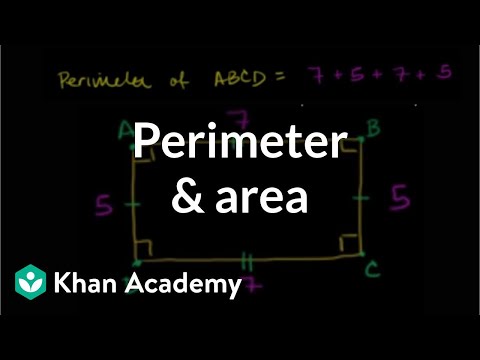
A broad set of tutorials covering perimeter area and volume with and without algebra. Perimeter and Area Basics. Area and Perimeter. Perimeter of a Polygon. Perimeter of a shape. Perimeter 1. Finding dimensions given perimeter. Area 1. Finding dimensions given area. Perimeter and Area Basics. Triangle Area Proofs. Area of triangles. Interesting Perimeter and Area Problems. Area of Diagonal Generated Triangles of Rectangle are Equal. Area of an equilateral triangle. Area of shaded region made from equilateral triangles. Shaded areas. Challenging Perimeter Problem. Triangle inqequality theorem. Triangle inequality theorem. Koch Snowflake Fractal. Area of an equilateral triangle. Area of Koch Snowflake (part 1) - Advanced. Area of Koch Snowflake (part 2) - Advanced. Heron's Formula. Heron's formula. Part 1 of Proof of Heron's Formula. Part 2 of the Proof of Heron's Formula. Circles: Radius, Diameter and Circumference. Parts of a Circle. Radius diameter and circumference. Area of a Circle. Area of a circle. Quadrilateral Overview. Quadrilateral Properties. Area of a Parallelogram. Area of parallelograms. Area of a trapezoid. Area of a kite. Area of trapezoids, rhombi, and kites. Perimeter of a Polygon. Perimeter and Area of a Non-Standard Polygon. How we measure volume. Measuring volume with unit cubes. Volume with unit cubes. Measuring volume as area times length. Volume of a rectangular prism or box examples. Volume 1. Volume word problem example. Volume word problems. Solid Geometry Volume. Cylinder Volume and Surface Area. Volume of a Sphere. Solid geometry. Perimeter and Area Basics. Area and Perimeter. Perimeter of a Polygon. Perimeter of a shape. Perimeter 1. Finding dimensions given perimeter. Area 1. Finding dimensions given area. Perimeter and Area Basics. Triangle Area Proofs. Area of triangles. Interesting Perimeter and Area Problems. Area of Diagonal Generated Triangles of Rectangle are Equal. Area of an equilateral triangle. Area of shaded region made from equilateral triangles. Shaded areas. Challenging Perimeter Problem. Triangle inqequality theorem. Triangle inequality theorem. Koch Snowflake Fractal. Area of an equilateral triangle. Area of Koch Snowflake (part 1) - Advanced. Area of Koch Snowflake (part 2) - Advanced. Heron's Formula. Heron's formula. Part 1 of Proof of Heron's Formula. Part 2 of the Proof of Heron's Formula. Circles: Radius, Diameter and Circumference. Parts of a Circle. Radius diameter and circumference. Area of a Circle. Area of a circle. Quadrilateral Overview. Quadrilateral Properties. Area of a Parallelogram. Area of parallelograms. Area of a trapezoid. Area of a kite. Area of trapezoids, rhombi, and kites. Perimeter of a Polygon. Perimeter and Area of a Non-Standard Polygon. How we measure volume. Measuring volume with unit cubes. Volume with unit cubes. Measuring volume as area times length. Volume of a rectangular prism or box examples. Volume 1. Volume word problem example. Volume word problems. Solid Geometry Volume. Cylinder Volume and Surface Area. Volume of a Sphere. Solid geometry.

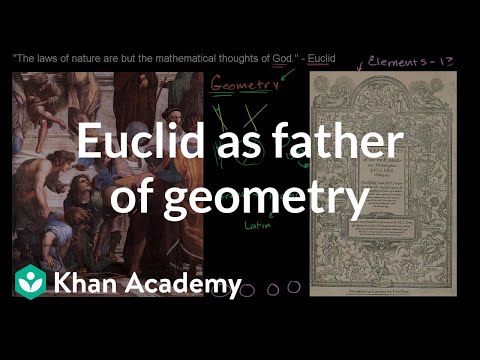
Understanding the purpose, notation, and building blocks of geometry. Euclid as the Father of Geometry. Language and Notation of Basic Geometry. Lines, Line Segments, and Rays. Identifying Rays. Identifying Parallel and Perpendicular Lines.

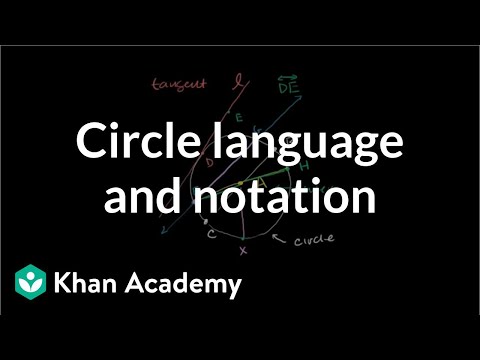
Finding measurements and applying and proving circle theorems. Language and Notation of the Circle. Circles: Radius, Diameter and Circumference. Parts of a Circle. Three Points Defining a Circle. Area of a Circle. Pi Is (still) Wrong.. Right Triangles Inscribed in Circles (Proof). Right Triangles Inscribed in Circles (Proof). Perpendicular Radius Bisects Chord. Incenter and incircles of a triangle. Inradius Perimeter and Area.

Conditional statements and deductive reasoning. Conditional statements exercise examples. Logical argument and deductive reasoning exercise example. Conditional statements and deductive reasoning. Conditional statements exercise examples. Logical argument and deductive reasoning exercise example.

Let's think more visually about things like shifts, rotations, scaling and symmetry. Axis of symmetry. Translations of polygons. Determining a translation for a shape. Rotation of polygons example. Axis of symmetry. Translations of polygons. Determining a translation for a shape. Rotation of polygons example.

Identifying types of quadrilaterals, finding measurements, and applying and proving postulates. Quadrilateral Overview. Quadrilateral Properties. Area of a Parallelogram. Area of a Regular Hexagon. Sum of Interior Angles of a Polygon. Sum of the exterior angles of convex polygon. Proof - Opposite Angles of Parallelogram Congruent. Proof - Opposite Sides of Parallelogram Congruent. Proof - Diagonals of a Parallelogram Bisect Each Other. Rhombus Diagonals. Proof - Rhombus Diagonals are Perpendicular Bisectors. Proof - Rhombus Area Half Product of Diagonal Length. Area of a Parallelogram. Area of a Regular Hexagon. Problem involving angle derived from square and circle. 2003 AIME II Problem 7. CA Geometry: Deducing Angle Measures.

Sal does the 80 problems from the released questions from the California Standards Test for Geometry. Basic understanding of Algebra I necessary. Interesting Perimeter and Area Problems. Challenging Perimeter Problem. CA Geometry: deductive reasoning. CA Geometry: Proof by Contradiction. CA Geometry: More Proofs. CA Geometry: Similar Triangles 1. CA Geometry: Similar Triangles 2. CA Geometry: More on congruent and similar triangles. CA Geometry: Triangles and Parallelograms. CA Geometry: Area, Pythagorean Theorem. CA Geometry: Area, Circumference, Volume. CA Geometry: Pythagorean Theorem, Area. CA Geometry: Exterior Angles. CA Geometry: Deducing Angle Measures. CA Geometry: Pythagorean Theorem, Compass Constructions. CA Geometry: Compass Construction. CA Geometry: Basic Trigonometry. CA Geometry: More Trig. CA Geometry: Circle Area Chords Tangent. CA Geometry: Secants and Translations. Interesting Perimeter and Area Problems. Challenging Perimeter Problem. CA Geometry: deductive reasoning. CA Geometry: Proof by Contradiction. CA Geometry: More Proofs. CA Geometry: Similar Triangles 1. CA Geometry: Similar Triangles 2. CA Geometry: More on congruent and similar triangles. CA Geometry: Triangles and Parallelograms. CA Geometry: Area, Pythagorean Theorem. CA Geometry: Area, Circumference, Volume. CA Geometry: Pythagorean Theorem, Area. CA Geometry: Exterior Angles. CA Geometry: Deducing Angle Measures. CA Geometry: Pythagorean Theorem, Compass Constructions. CA Geometry: Compass Construction. CA Geometry: Basic Trigonometry. CA Geometry: More Trig. CA Geometry: Circle Area Chords Tangent. CA Geometry: Secants and Translations.

This topic introduces the basic conceptual tools that underpin our journey through Euclidean geometry. These include the ideas of points, lines, line segments, rays, and planes. Euclid as the Father of Geometry. Language and Notation of Basic Geometry. Lines, Line Segments, and Rays. Recognizing rays lines and line segments. Specifying planes in three dimensions. Points, lines, and planes. Language and Notation of the Circle. The Golden Ratio. Identifying Rays. Measuring segments. Measuring segments. Congruent segments. Congruent segments. Segment addition. Segment addition. Algebraic midpoint of a segment exercise. Midpoint of a segment. Euclid as the Father of Geometry. Language and Notation of Basic Geometry. Lines, Line Segments, and Rays. Recognizing rays lines and line segments. Specifying planes in three dimensions. Points, lines, and planes. Language and Notation of the Circle. The Golden Ratio. Identifying Rays. Measuring segments. Measuring segments. Congruent segments. Congruent segments. Segment addition. Segment addition. Algebraic midpoint of a segment exercise. Midpoint of a segment.

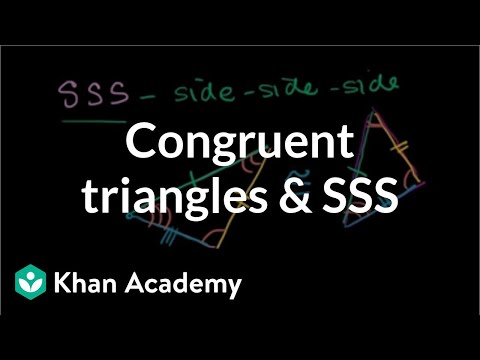
If you can take one figure and flip, shift and rotate (not resize) it to be identical to another figure, then the two figures are congruent. This topic explores this foundational idea in geometry. Congruent Triangles and SSS. SSS to Show a Radius is Perpendicular to a Chord that it Bisects. Other Triangle Congruence Postulates. Two column proof showing segments are perpendicular. Finding Congruent Triangles. Congruency postulates. More on why SSA is not a postulate. Perpendicular Radius Bisects Chord. Congruent Triangle Proof Example. Congruent Triangle Example 2. Congruent triangles 1. Congruent triangles 2. Congruent legs and base angles of Isosceles Triangles. Equilateral Triangle Sides and Angles Congruent. Equilateral and Isosceles Example Problems. Triangle types. Triangle angles 1. Another Isosceles Example Problem. Example involving an isosceles triangle and parallel lines. Figuring out all the angles for congruent triangles example. Basic Triangle Proofs Module Example. Basic Triangle Proofs Module Example 2. Basic triangle proofs. Fill-in-the-blank triangle proofs example 1. Fill-in-the-blank triangle proofs example 2. Fill-in-the-blank triangle proofs. Wrong statements in triangle proofs example 1. Wrong statements in triangle proofs. Problem involving angle derived from square and circle. Congruent Triangles and SSS. SSS to Show a Radius is Perpendicular to a Chord that it Bisects. Other Triangle Congruence Postulates. Two column proof showing segments are perpendicular. Finding Congruent Triangles. Congruency postulates. More on why SSA is not a postulate. Perpendicular Radius Bisects Chord. Congruent Triangle Proof Example. Congruent Triangle Example 2. Congruent triangles 1. Congruent triangles 2. Congruent legs and base angles of Isosceles Triangles. Equilateral Triangle Sides and Angles Congruent. Equilateral and Isosceles Example Problems. Triangle types. Triangle angles 1. Another Isosceles Example Problem. Example involving an isosceles triangle and parallel lines. Figuring out all the angles for congruent triangles example. Basic Triangle Proofs Module Example. Basic Triangle Proofs Module Example 2. Basic triangle proofs. Fill-in-the-blank triangle proofs example 1. Fill-in-the-blank triangle proofs example 2. Fill-in-the-blank triangle proofs. Wrong statements in triangle proofs example 1. Wrong statements in triangle proofs. Problem involving angle derived from square and circle.

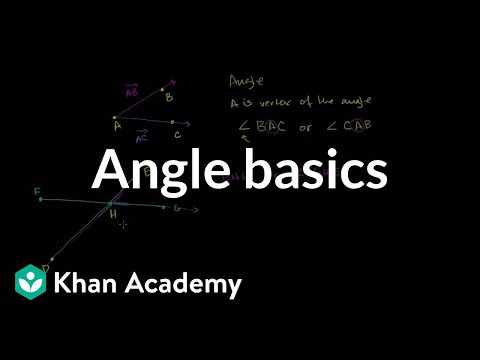
This topic continues our journey through the world of Euclid by helping us understand angles and how they can relate to each other. Angle basics. Measuring angles in degrees. Using a protractor. Measuring angles. Measuring angles. Acute right and obtuse angles. Angle types. Vertical, adjacent and linearly paired angles. Exploring angle pairs. Introduction to vertical angles. Vertical angles. Using algebra to find the measures of vertical angles. Vertical angles 2. Proof-Vertical Angles are Equal. Angles Formed by Parallel Lines and Transversals. Identifying Parallel and Perpendicular Lines. Figuring out angles between transversal and parallel lines. Congruent angles. Parallel lines 1. Using algebra to find measures of angles formed from transversal. Parallel lines 2. CA Geometry: Deducing Angle Measures. Proof - Sum of Measures of Angles in a Triangle are 180. Triangle Angle Example 1. Triangle Angle Example 2. Triangle Angle Example 3. Challenging Triangle Angle Problem. Proof - Corresponding Angle Equivalence Implies Parallel Lines. Finding more angles. Angles 1. Angles 2. Sum of Interior Angles of a Polygon. Angles of a polygon. Sum of the exterior angles of convex polygon. Introduction to angles (old). Angles (part 2). Angles (part 3). Angles formed between transversals and parallel lines. Angles of parallel lines 2. The Angle Game. Angle Game (part 2). Acute right and obtuse angles. Complementary and supplementary angles. Complementary and supplementary angles. Example using algebra to find measure of complementary angles. Example using algebra to find measure of supplementary angles. Angle addition postulate. Angle basics. Measuring angles in degrees. Using a protractor. Measuring angles. Measuring angles. Acute right and obtuse angles. Angle types. Vertical, adjacent and linearly paired angles. Exploring angle pairs. Introduction to vertical angles. Vertical angles. Using algebra to find the measures of vertical angles. Vertical angles 2. Proof-Vertical Angles are Equal. Angles Formed by Parallel Lines and Transversals. Identifying Parallel and Perpendicular Lines. Figuring out angles between transversal and parallel lines. Congruent angles. Parallel lines 1. Using algebra to find measures of angles formed from transversal. Parallel lines 2. CA Geometry: Deducing Angle Measures. Proof - Sum of Measures of Angles in a Triangle are 180. Triangle Angle Example 1. Triangle Angle Example 2. Triangle Angle Example 3. Challenging Triangle Angle Problem. Proof - Corresponding Angle Equivalence Implies Parallel Lines. Finding more angles. Angles 1. Angles 2. Sum of Interior Angles of a Polygon. Angles of a polygon. Sum of the exterior angles of convex polygon. Introduction to angles (old). Angles (part 2). Angles (part 3). Angles formed between transversals and parallel lines. Angles of parallel lines 2. The Angle Game. Angle Game (part 2). Acute right and obtuse angles. Complementary and supplementary angles. Complementary and supplementary angles. Example using algebra to find measure of complementary angles. Example using algebra to find measure of supplementary angles. Angle addition postulate.

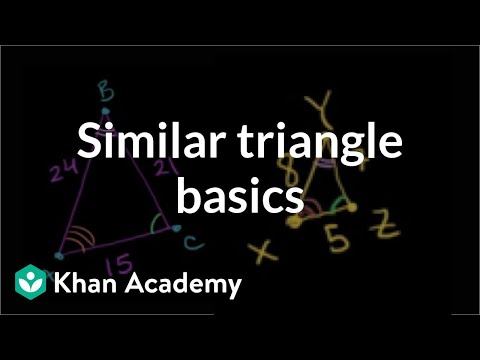
Similar Triangle Basics. Similarity Postulates. Similar triangles 1. Similar Triangle Example Problems. Similar triangles 2. Similarity Example Problems. Solving similar triangles 1. Similarity example where same side plays different roles. Challenging Similarity Problem. Finding Area Using Similarity and Congruence. Similar triangles. Similar triangles (part 2). Similar Triangle Basics. Similarity Postulates. Similar triangles 1. Similar Triangle Example Problems. Similar triangles 2. Similarity Example Problems. Solving similar triangles 1. Similarity example where same side plays different roles. Challenging Similarity Problem. Finding Area Using Similarity and Congruence. Similar triangles. Similar triangles (part 2).

Triangles are not always right (although they are never wrong), but when they are it opens up an exciting world of possibilities. Not only are right triangles cool in their own right (pun intended), they are the basis of very important ideas in analytic geometry (the distance between two points in space) and trigonometry. Pythagorean Theorem. The Pythagorean theorem intro. Pythagorean Theorem 1. Pythagorean Theorem 2. Pythagorean Theorem 3. Pythagorean theorem. Introduction to the Pythagorean Theorem. Pythagorean Theorem II. Garfield's proof of the Pythagorean Theorem. Bhaskara's proof of Pythagorean Theorem. Pythagorean Theorem Proof Using Similarity. Another Pythagorean Theorem Proof. 30-60-90 Triangle Side Ratios Proof. 45-45-90 Triangle Side Ratios. 30-60-90 Triangle Example Problem. Special right triangles. Area of a Regular Hexagon. 45-45-90 Triangles. Intro to 30-60-90 Triangles. 30-60-90 Triangles II. Pythagorean Theorem. The Pythagorean theorem intro. Pythagorean Theorem 1. Pythagorean Theorem 2. Pythagorean Theorem 3. Pythagorean theorem. Introduction to the Pythagorean Theorem. Pythagorean Theorem II. Garfield's proof of the Pythagorean Theorem. Bhaskara's proof of Pythagorean Theorem. Pythagorean Theorem Proof Using Similarity. Another Pythagorean Theorem Proof. 30-60-90 Triangle Side Ratios Proof. 45-45-90 Triangle Side Ratios. 30-60-90 Triangle Example Problem. Special right triangles. Area of a Regular Hexagon. 45-45-90 Triangles. Intro to 30-60-90 Triangles. 30-60-90 Triangles II.

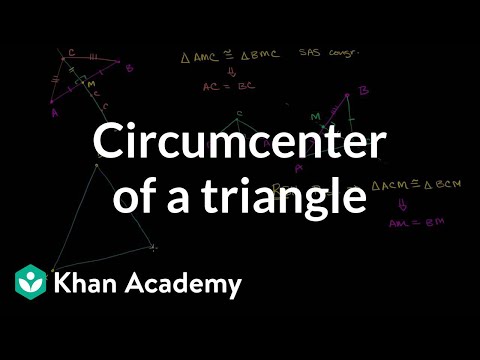
You probably like triangles. You think they are useful. They show up a lot. What you'll see in this topic is that they are far more magical and mystical than you ever imagined!. Circumcenter of a Triangle. Circumcenter of a Right Triangle. Three Points Defining a Circle. Area Circumradius Formula Proof. 2003 AIME II Problem 7. Point Line Distance and Angle Bisectors. Incenter and incircles of a triangle. Inradius Perimeter and Area. Angle Bisector Theorem Proof. Angle Bisector Theorem Examples. Angle bisector theorem. Triangle Medians and Centroids. Triangle Medians and Centroids (2D Proof). Medians divide into smaller triangles of equal area. Exploring Medial Triangles. Proving that the Centroid is 2-3rds along the Median. Median Centroid Right Triangle Example. Proof - Triangle Altitudes are Concurrent (Orthocenter). Common Orthocenter and Centroid. Review of Triangle Properties. Euler Line. Euler's Line Proof. Constructing a perpendicular bisector using a compass and straightedge. Constructing a perpendicular line using a compass and straightedge. Constructing an angle bisector using a compass and straightedge. Compass Constructions. Circumcenter of a Triangle. Circumcenter of a Right Triangle. Three Points Defining a Circle. Area Circumradius Formula Proof. 2003 AIME II Problem 7. Point Line Distance and Angle Bisectors. Incenter and incircles of a triangle. Inradius Perimeter and Area. Angle Bisector Theorem Proof. Angle Bisector Theorem Examples. Angle bisector theorem. Triangle Medians and Centroids. Triangle Medians and Centroids (2D Proof). Medians divide into smaller triangles of equal area. Exploring Medial Triangles. Proving that the Centroid is 2-3rds along the Median. Median Centroid Right Triangle Example. Proof - Triangle Altitudes are Concurrent (Orthocenter). Common Orthocenter and Centroid. Review of Triangle Properties. Euler Line. Euler's Line Proof. Constructing a perpendicular bisector using a compass and straightedge. Constructing a perpendicular line using a compass and straightedge. Constructing an angle bisector using a compass and straightedge. Compass Constructions.

Not all things with four sides have to be squares or rectangles! We will now broaden our understanding of quadrilaterals!. Quadrilateral Overview. Quadrilateral Properties. Proof - Opposite Sides of Parallelogram Congruent. Proof - Diagonals of a Parallelogram Bisect Each Other. Proof - Opposite Angles of Parallelogram Congruent. Proof - Rhombus Diagonals are Perpendicular Bisectors. Proof - Rhombus Area Half Product of Diagonal Length. Area of a Parallelogram. Whether a Special Quadrilateral Can Exist. Rhombus Diagonals. Quadrilateral Overview. Quadrilateral Properties. Proof - Opposite Sides of Parallelogram Congruent. Proof - Diagonals of a Parallelogram Bisect Each Other. Proof - Opposite Angles of Parallelogram Congruent. Proof - Rhombus Diagonals are Perpendicular Bisectors. Proof - Rhombus Area Half Product of Diagonal Length. Area of a Parallelogram. Whether a Special Quadrilateral Can Exist. Rhombus Diagonals.

Language and Notation of the Circle. Circles: Radius, Diameter and Circumference. Length of an arc that subtends a central angle. Finding central angle measure given arc length. Parts of a Circle. Area of a Circle. Area of a sector given a central angle. Inscribed and Central Angles. Perpendicular Radius Bisects Chord. Right Triangles Inscribed in Circles (Proof). Area of Inscribed Equilateral Triangle (some basic trig used). Language and Notation of the Circle. Circles: Radius, Diameter and Circumference. Length of an arc that subtends a central angle. Finding central angle measure given arc length. Parts of a Circle. Area of a Circle. Area of a sector given a central angle. Inscribed and Central Angles. Perpendicular Radius Bisects Chord. Right Triangles Inscribed in Circles (Proof). Area of Inscribed Equilateral Triangle (some basic trig used).