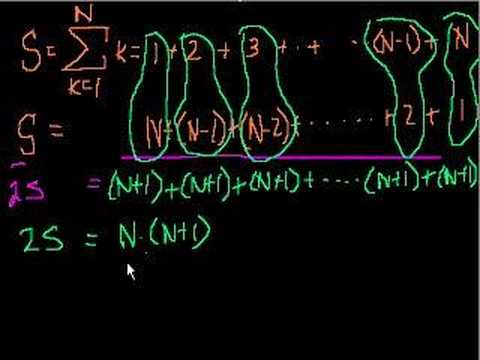Online courses directory (381)

Limit introduction, squeeze theorem, and epsilon-delta definition of limits. Introduction to limits. Limit at a point of discontinuity. Determining which limit statements are true. Limit properties. Limit example 1. Limits 1. One-sided limits from graphs. One-sided limits from graphs. Introduction to Limits. Limit Examples (part 1). Limit Examples (part 2). Limit Examples (part 3). Limit Examples w/ brain malfunction on first prob (part 4). More Limits. Limits 1. Limits and infinity. Limits at positive and negative infinity. More limits at infinity. Limits with two horizontal asymptotes. Limits 2. Squeeze Theorem. Proof: lim (sin x)/x. Limit intuition review. Building the idea of epsilon-delta definition. Epsilon-delta definition of limits. Proving a limit using epsilon-delta definition. Limits to define continuity. Continuity. Epsilon Delta Limit Definition 1. Epsilon Delta Limit Definition 2. Introduction to limits. Limit at a point of discontinuity. Determining which limit statements are true. Limit properties. Limit example 1. Limits 1. One-sided limits from graphs. One-sided limits from graphs. Introduction to Limits. Limit Examples (part 1). Limit Examples (part 2). Limit Examples (part 3). Limit Examples w/ brain malfunction on first prob (part 4). More Limits. Limits 1. Limits and infinity. Limits at positive and negative infinity. More limits at infinity. Limits with two horizontal asymptotes. Limits 2. Squeeze Theorem. Proof: lim (sin x)/x. Limit intuition review. Building the idea of epsilon-delta definition. Epsilon-delta definition of limits. Proving a limit using epsilon-delta definition. Limits to define continuity. Continuity. Epsilon Delta Limit Definition 1. Epsilon Delta Limit Definition 2.

Line integral of scalar and vector-valued functions. Green's theorem and 2-D divergence theorem. Introduction to the Line Integral. Line Integral Example 1. Line Integral Example 2 (part 1). Line Integral Example 2 (part 2). Position Vector Valued Functions. Derivative of a position vector valued function. Differential of a vector valued function. Vector valued function derivative example. Line Integrals and Vector Fields. Using a line integral to find the work done by a vector field example. Parametrization of a Reverse Path. Scalar Field Line Integral Independent of Path Direction. Vector Field Line Integrals Dependent on Path Direction. Path Independence for Line Integrals. Closed Curve Line Integrals of Conservative Vector Fields. Example of Closed Line Integral of Conservative Field. Second Example of Line Integral of Conservative Vector Field. Green's Theorem Proof Part 1. Green's Theorem Proof (part 2). Green's Theorem Example 1. Green's Theorem Example 2. Constructing a unit normal vector to a curve. 2 D Divergence Theorem. Conceptual clarification for 2-D Divergence Theorem. Introduction to the Line Integral. Line Integral Example 1. Line Integral Example 2 (part 1). Line Integral Example 2 (part 2). Position Vector Valued Functions. Derivative of a position vector valued function. Differential of a vector valued function. Vector valued function derivative example. Line Integrals and Vector Fields. Using a line integral to find the work done by a vector field example. Parametrization of a Reverse Path. Scalar Field Line Integral Independent of Path Direction. Vector Field Line Integrals Dependent on Path Direction. Path Independence for Line Integrals. Closed Curve Line Integrals of Conservative Vector Fields. Example of Closed Line Integral of Conservative Field. Second Example of Line Integral of Conservative Vector Field. Green's Theorem Proof Part 1. Green's Theorem Proof (part 2). Green's Theorem Example 1. Green's Theorem Example 2. Constructing a unit normal vector to a curve. 2 D Divergence Theorem. Conceptual clarification for 2-D Divergence Theorem.

Thinking about forms of derivatives in multi-dimensions and for vector-valued functions: partial derivatives, gradient, divergence and curl. Partial Derivatives. Partial Derivatives 2. Gradient 1. Gradient of a scalar field. Divergence 1. Divergence 2. Divergence 3. Curl 1. Curl 2. Curl 3. Partial Derivatives. Partial Derivatives 2. Gradient 1. Gradient of a scalar field. Divergence 1. Divergence 2. Divergence 3. Curl 1. Curl 2. Curl 3.

Sequences, series and approximating functions. Maclaurin and Taylor series. Sequences and Series (part 1). Sequences and series (part 2). Maclaurin and Taylor Series Intuition. Cosine Taylor Series at 0 (Maclaurin). Sine Taylor Series at 0 (Maclaurin). Taylor Series at 0 (Maclaurin) for e to the x. Euler's Formula and Euler's Identity. Visualizing Taylor Series Approximations. Generalized Taylor Series Approximation. Visualizing Taylor Series for e^x. Error or Remainder of a Taylor Polynomial Approximation. Proof: Bounding the Error or Remainder of a Taylor Polynomial Approximation. Polynomial approximation of functions (part 1). Polynomial approximation of functions (part 2). Approximating functions with polynomials (part 3). Polynomial approximation of functions (part 4). Polynomial approximations of functions (part 5). Polynomial approximation of functions (part 6). Polynomial approximation of functions (part 7). Taylor Polynomials. Sequences and Series (part 1). Sequences and series (part 2). Maclaurin and Taylor Series Intuition. Cosine Taylor Series at 0 (Maclaurin). Sine Taylor Series at 0 (Maclaurin). Taylor Series at 0 (Maclaurin) for e to the x. Euler's Formula and Euler's Identity. Visualizing Taylor Series Approximations. Generalized Taylor Series Approximation. Visualizing Taylor Series for e^x. Error or Remainder of a Taylor Polynomial Approximation. Proof: Bounding the Error or Remainder of a Taylor Polynomial Approximation. Polynomial approximation of functions (part 1). Polynomial approximation of functions (part 2). Approximating functions with polynomials (part 3). Polynomial approximation of functions (part 4). Polynomial approximations of functions (part 5). Polynomial approximation of functions (part 6). Polynomial approximation of functions (part 7). Taylor Polynomials.

Using definite integrals with the shell and disc methods to find volumes of solids of revolution. Disk method around x-axis. Generalizing disc method around x-axis. Disc method around y-axis. Disc method (washer method) for rotation around x-axis. Generalizing the washer method. Disc method rotation around horizontal line. Washer method rotating around non-axis. Part 2 of washer for non axis rotation. Disc method rotating around vertical line. Calculating integral disc method around vertical line. Washer or ring method for vertical line rotation. Evaluating integral for washer method around vertical line. Shell method for rotating around vertical line. Evaluating integral for shell method example. Shell method for rotating around horizontal line. Shell method with two functions of x. Calculating integral with shell method. Shell method with two functions of y. Part 2 of shell method with 2 functions of y. Disc method: function rotated about x-axis. Disc method (rotating f(x) about x axis). Volume of a sphere. Disc method with outer and inner function boundaries. Shell method to rotate around y-axis. Disk method: rotating x=f(y) around the y-axis. Shell method around a non-axis line. Shell method around a non-axis line 2. Disk method around x-axis. Generalizing disc method around x-axis. Disc method around y-axis. Disc method (washer method) for rotation around x-axis. Generalizing the washer method. Disc method rotation around horizontal line. Washer method rotating around non-axis. Part 2 of washer for non axis rotation. Disc method rotating around vertical line. Calculating integral disc method around vertical line. Washer or ring method for vertical line rotation. Evaluating integral for washer method around vertical line. Shell method for rotating around vertical line. Evaluating integral for shell method example. Shell method for rotating around horizontal line. Shell method with two functions of x. Calculating integral with shell method. Shell method with two functions of y. Part 2 of shell method with 2 functions of y. Disc method: function rotated about x-axis. Disc method (rotating f(x) about x axis). Volume of a sphere. Disc method with outer and inner function boundaries. Shell method to rotate around y-axis. Disk method: rotating x=f(y) around the y-axis. Shell method around a non-axis line. Shell method around a non-axis line 2.

Parameterizing a surface. Surface integrals. Stokes' theorem. Introduction to Parametrizing a Surface with Two Parameters. Determining a Position Vector-Valued Function for a Parametrization of Two Parameters. Partial Derivatives of Vector-Valued Functions. Introduction to the Surface Integral. Example of calculating a surface integral part 1. Example of calculating a surface integral part 2. Example of calculating a surface integral part 3. Surface Integral Example Part 1 - Parameterizing the Unit Sphere. Surface Integral Example Part 2 - Calculating the Surface Differential. Surface Integral Example Part 3 - The Home Stretch. Surface Integral Ex2 part 1 - Parameterizing the Surface. Surface Integral Ex2 part 2 - Evaluating Integral. Surface Integral Ex3 part 1 - Parameterizing the Outside Surface. Surface Integral Ex3 part 2 - Evaluating the Outside Surface. Surface Integral Ex3 part 3 - Top surface. Surface Integral Ex3 part 4 - Home Stretch. Conceptual Understanding of Flux in Three Dimensions. Constructing a unit normal vector to a surface. Vector representation of a Surface Integral. Stokes' Theorem Intuition. Green's and Stokes' Theorem Relationship. Orienting Boundary with Surface. Orientation and Stokes. Conditions for Stokes Theorem. Stokes Example Part 1. Part 2 Parameterizing the Surface. Stokes Example Part 3 - Surface to Double Integral. Stokes Example Part 4 - Curl and Final Answer. Evaluating Line Integral Directly - Part 1. Evaluating Line Integral Directly - Part 2. Stokes' Theorem Proof Part 1. Stokes' Theorem Proof Part 2. Stokes' Theorem Proof Part 3. Stokes' Theorem Proof Part 4. Stokes' Theorem Proof Part 5. Stokes' Theorem Proof Part 6. Stokes' Theorem Proof Part 7. Introduction to Parametrizing a Surface with Two Parameters. Determining a Position Vector-Valued Function for a Parametrization of Two Parameters. Partial Derivatives of Vector-Valued Functions. Introduction to the Surface Integral. Example of calculating a surface integral part 1. Example of calculating a surface integral part 2. Example of calculating a surface integral part 3. Surface Integral Example Part 1 - Parameterizing the Unit Sphere. Surface Integral Example Part 2 - Calculating the Surface Differential. Surface Integral Example Part 3 - The Home Stretch. Surface Integral Ex2 part 1 - Parameterizing the Surface. Surface Integral Ex2 part 2 - Evaluating Integral. Surface Integral Ex3 part 1 - Parameterizing the Outside Surface. Surface Integral Ex3 part 2 - Evaluating the Outside Surface. Surface Integral Ex3 part 3 - Top surface. Surface Integral Ex3 part 4 - Home Stretch. Conceptual Understanding of Flux in Three Dimensions. Constructing a unit normal vector to a surface. Vector representation of a Surface Integral. Stokes' Theorem Intuition. Green's and Stokes' Theorem Relationship. Orienting Boundary with Surface. Orientation and Stokes. Conditions for Stokes Theorem. Stokes Example Part 1. Part 2 Parameterizing the Surface. Stokes Example Part 3 - Surface to Double Integral. Stokes Example Part 4 - Curl and Final Answer. Evaluating Line Integral Directly - Part 1. Evaluating Line Integral Directly - Part 2. Stokes' Theorem Proof Part 1. Stokes' Theorem Proof Part 2. Stokes' Theorem Proof Part 3. Stokes' Theorem Proof Part 4. Stokes' Theorem Proof Part 5. Stokes' Theorem Proof Part 6. Stokes' Theorem Proof Part 7.

Calculating derivatives. Power rule. Product and quotient rules. Chain Rule. Implicit differentiation. Derivatives of common functions. Newton Leibniz and Usain Bolt. Slope of a line secant to a curve. Slope of a secant line example 1. Slope of a secant line example 2. Slope of a secant line example 3. Approximating instantaneous rate of change word problem. Approximating equation of tangent line word problem. Slope of secant lines. Derivative as slope of a tangent line. Tangent slope as limiting value of secant slope example 1. Tangent slope as limiting value of secant slope example 2. Tangent slope as limiting value of secant slope example 3. Tangent slope is limiting value of secant slope. Calculating slope of tangent line using derivative definition. Derivatives 1. The derivative of f(x)=x^2 for any x. Formal and alternate form of the derivative. Formal and alternate form of the derivative for ln x. Formal and alternate form of the derivative example 1. The formal and alternate form of the derivative. Interpreting slope of a curve exercise. Recognizing slope of curves. Calculus: Derivatives 1. Calculus: Derivatives 2. Derivative Intuition Module. Derivative intuition. Graphs of functions and their derivatives example 1. Where a function is not differentiable. Identifying a function's derivative example. Figuring out which function is the the derivative. Graphs of functions and their derivatives. Intuitively drawing the derivative of a function. Intuitively drawing the antiderivative of a function. Visualizing derivatives exercise. Visualizing derivatives. Power Rule. Is the power rule reasonable. Derivative properties and polynomial derivatives. Power rule. Proof: d/dx(x^n). Proof: d/dx(sqrt(x)). Power rule introduction. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Chain rule introduction. Chain rule definition and example. Chain rule with triple composition. Chain rule for derivative of 2^x. Derivative of log with arbitrary base. Chain rule 1. Extreme Derivative Word Problem (advanced). The Chain Rule. Chain Rule Examples. Even More Chain Rule. More examples using multiple rules. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Applying the product rule for derivatives. Product rule for more than two functions. Product rule. Quotient rule from product rule. Quotient rule for derivative of tan x. Quotient rule. Using the product rule and the chain rule. Product Rule. Quotient rule and common derivatives. Equation of a tangent line. Implicit differentiation. Showing explicit and implicit differentiation give same result. Implicit derivative of (x-y)^2 = x + y + 1. Implicit derivative of y = cos(5x - 3y). Implicit derivative of (x^2+y^2)^3 = 5x^2y^2. Finding slope of tangent line with implicit differentiation. Implicit derivative of e^(xy^2) = x - y. Derivative of x^(x^x). Implicit differentiation. Proof: d/dx(ln x) = 1/x. Proof: d/dx(e^x) = e^x. Proofs of derivatives of ln(x) and e^x. Newton Leibniz and Usain Bolt. Slope of a line secant to a curve. Slope of a secant line example 1. Slope of a secant line example 2. Slope of a secant line example 3. Approximating instantaneous rate of change word problem. Approximating equation of tangent line word problem. Slope of secant lines. Derivative as slope of a tangent line. Tangent slope as limiting value of secant slope example 1. Tangent slope as limiting value of secant slope example 2. Tangent slope as limiting value of secant slope example 3. Tangent slope is limiting value of secant slope. Calculating slope of tangent line using derivative definition. Derivatives 1. The derivative of f(x)=x^2 for any x. Formal and alternate form of the derivative. Formal and alternate form of the derivative for ln x. Formal and alternate form of the derivative example 1. The formal and alternate form of the derivative. Interpreting slope of a curve exercise. Recognizing slope of curves. Calculus: Derivatives 1. Calculus: Derivatives 2. Derivative Intuition Module. Derivative intuition. Graphs of functions and their derivatives example 1. Where a function is not differentiable. Identifying a function's derivative example. Figuring out which function is the the derivative. Graphs of functions and their derivatives. Intuitively drawing the derivative of a function. Intuitively drawing the antiderivative of a function. Visualizing derivatives exercise. Visualizing derivatives. Power Rule. Is the power rule reasonable. Derivative properties and polynomial derivatives. Power rule. Proof: d/dx(x^n). Proof: d/dx(sqrt(x)). Power rule introduction. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Chain rule introduction. Chain rule definition and example. Chain rule with triple composition. Chain rule for derivative of 2^x. Derivative of log with arbitrary base. Chain rule 1. Extreme Derivative Word Problem (advanced). The Chain Rule. Chain Rule Examples. Even More Chain Rule. More examples using multiple rules. Derivatives of sin x, cos x, tan x, e^x and ln x. Special derivatives. Applying the product rule for derivatives. Product rule for more than two functions. Product rule. Quotient rule from product rule. Quotient rule for derivative of tan x. Quotient rule. Using the product rule and the chain rule. Product Rule. Quotient rule and common derivatives. Equation of a tangent line. Implicit differentiation. Showing explicit and implicit differentiation give same result. Implicit derivative of (x-y)^2 = x + y + 1. Implicit derivative of y = cos(5x - 3y). Implicit derivative of (x^2+y^2)^3 = 5x^2y^2. Finding slope of tangent line with implicit differentiation. Implicit derivative of e^(xy^2) = x - y. Derivative of x^(x^x). Implicit differentiation. Proof: d/dx(ln x) = 1/x. Proof: d/dx(e^x) = e^x. Proofs of derivatives of ln(x) and e^x.

CA Algebra I: Number Properties and Absolute Value. CA Algebra I: Simplifying Expressions. CA Algebra I: Simple Logical Arguments. CA Algebra I: Graphing Inequalities. CA Algebra I: Slope and Y-intercept. CA Algebra I: Systems of Inequalities. CA Algebra I: Simplifying Expressions. CA Algebra I: Factoring Quadratics. CA Algebra I: Completing the Square. CA Algebra I: Quadratic Equation. CA Algebra I: Quadratic Roots. CA Algebra I: Rational Expressions 1. CA Algebra I: Rational Expressions 2. CA Algebra I: Word Problems. CA Algebra I: More Word Problems. CA Algebra I: Functions.

California Standards Test: Algebra II. California Standards Test: Algebra II (Graphing Inequalities). CA Standards: Algebra II (Algebraic Division/Multiplication). CA Standards: Algebra II. Algebra II: Simplifying Polynomials. Algebra II: Imaginary and Complex Numbers. Algebra II: Complex numbers and conjugates. Algebra II: Quadratics and Shifts. Examples: Graphing and interpreting quadratics. Hyperbola and parabola examples. Algebra II: Circles and Logarithms. Algebra II: Logarithms Exponential Growth. Algebra II: Logarithms and more. Algebra II: Functions, Combinatorics. Algebra II: binomial Expansion and Combinatorics. Algebra II: Binomial Expansions, Geometric Series Sum. Algebra II: Functions and Probability. Algebra II: Probability and Statistics. Algebra II: Mean and Standard Deviation.

CA Geometry: deductive reasoning. CA Geometry: Proof by Contradiction. CA Geometry: More Proofs. CA Geometry: Similar Triangles 1. CA Geometry: Similar Triangles 2. CA Geometry: More on congruent and similar triangles. CA Geometry: Triangles and Parallelograms. CA Geometry: Area, Pythagorean Theorem. CA Geometry: Area, Circumference, Volume. CA Geometry: Pythagorean Theorem, Area. CA Geometry: Exterior Angles. CA Geometry: Deducing Angle Measures. CA Geometry: Pythagorean Theorem, Compass Constructions. CA Geometry: Compass Construction. CA Geometry: Basic Trigonometry. CA Geometry: More Trig. CA Geometry: Circle Area Chords Tangent. CA Geometry: Secants and Translations.

Acid Base Introduction. pH, pOH of Strong Acids and Bases. pH of a Weak Acid. pH of a Weak Base. Conjugate Acids and Bases. pKa and pKb Relationship. Buffers and Hendersen-Hasselbalch. Strong Acid Titration. Weak Acid Titration. Half Equivalence Point. Titration Roundup. Acid Base Titration. Acid Base Introduction. pH, pOH of Strong Acids and Bases. pH of a Weak Acid. pH of a Weak Base. Conjugate Acids and Bases. pKa and pKb Relationship. Buffers and Hendersen-Hasselbalch. Strong Acid Titration. Weak Acid Titration. Half Equivalence Point. Titration Roundup. Acid Base Titration.

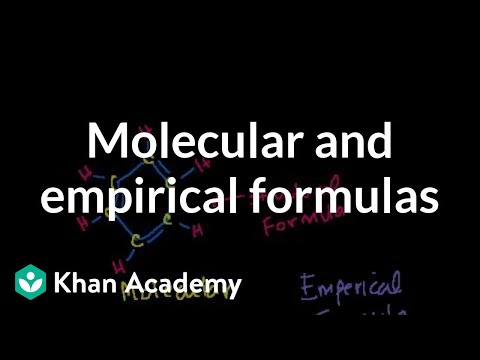
Molecular and Empirical Formulas. The Mole and Avogadro's Number. Formula from Mass Composition. Another mass composition problem. Balancing Chemical Equations. Stoichiometry. Stoichiometry Example Problem 1. Stoichiometry Example Problem 2. Stoichiometry: Limiting Reagent. Limiting Reactant Example Problem 1. Spectrophotometry Introduction. Spectrophotometry Example. Molecular and Empirical Formulas. The Mole and Avogadro's Number. Formula from Mass Composition. Another mass composition problem. Balancing Chemical Equations. Stoichiometry. Stoichiometry Example Problem 1. Stoichiometry Example Problem 2. Stoichiometry: Limiting Reagent. Limiting Reactant Example Problem 1. Spectrophotometry Introduction. Spectrophotometry Example.

Ideal Gas Equation: PV=nRT. Ideal Gas Equation Example 1. Ideal Gas Equation Example 2. Ideal Gas Equation Example 3. Ideal Gas Equation Example 4. Partial Pressure. Vapor Pressure Example. Ideal Gas Equation: PV=nRT. Ideal Gas Equation Example 1. Ideal Gas Equation Example 2. Ideal Gas Equation Example 3. Ideal Gas Equation Example 4. Partial Pressure. Vapor Pressure Example.

Elements and Atoms. Introduction to the atom. Elements and Atoms. Introduction to the atom.

Orbitals. More on orbitals and electron configuration. Electron configurations. Electron configurations 2. Valence Electrons. Orbitals. More on orbitals and electron configuration. Electron configurations. Electron configurations 2. Valence Electrons.

Introduction to Oxidation States. More on Oxidation States. Hydrogen Peroxide Correction. Redox Reactions. Galvanic Cells. Introduction to Oxidation States. More on Oxidation States. Hydrogen Peroxide Correction. Redox Reactions. Galvanic Cells.

Groups of the Periodic Table. Valence Electrons. Periodic Table Trends: Ionization Energy. Other Periodic Table Trends. Ionic, Covalent, and Metallic Bonds. Groups of the Periodic Table. Valence Electrons. Periodic Table Trends: Ionization Energy. Other Periodic Table Trends. Ionic, Covalent, and Metallic Bonds.

Types of Decay. Half-Life. Exponential Decay Formula Proof (can skip, involves Calculus). Introduction to Exponential Decay. More Exponential Decay Examples. Types of Decay. Half-Life. Exponential Decay Formula Proof (can skip, involves Calculus). Introduction to Exponential Decay. More Exponential Decay Examples.

Introduction to Kinetics. Reactions in Equilibrium. Mini-Video on Ion Size. Keq Intuition (mathy and not necessary to progress). Keq derivation intuition (can skip; bit mathy). Heterogeneous Equilibrium. Le Chatelier's Principle. Introduction to pH, pOH, and pKw. Introduction to Kinetics. Reactions in Equilibrium. Mini-Video on Ion Size. Keq Intuition (mathy and not necessary to progress). Keq derivation intuition (can skip; bit mathy). Heterogeneous Equilibrium. Le Chatelier's Principle. Introduction to pH, pOH, and pKw.

States of Matter. States of Matter Follow-Up. Specific Heat, Heat of Fusion and Vaporization. Chilling Water Problem. Phase Diagrams. Van Der Waals Forces. Covalent Networks, Metallic, and Ionic Crystals. Vapor Pressure. Suspensions, Colloids and Solutions. Solubility. Boiling Point Elevation and Freezing Point Suppression. Change of State Example. States of Matter. States of Matter Follow-Up. Specific Heat, Heat of Fusion and Vaporization. Chilling Water Problem. Phase Diagrams. Van Der Waals Forces. Covalent Networks, Metallic, and Ionic Crystals. Vapor Pressure. Suspensions, Colloids and Solutions. Solubility. Boiling Point Elevation and Freezing Point Suppression. Change of State Example.